Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
Review
. 2021 May 6:50:245-265.
doi: 10.1146/annurev-biophys-102220-083020. Epub 2021 Feb 19.

Analysis of Tandem Repeat Protein Folding Using Nearest-Neighbor Models

Mark Petersen  1   2 , Doug Barrick  2
Affiliations
Review

Analysis of Tandem Repeat Protein Folding Using Nearest-Neighbor Models

Mark Petersen et al. Annu Rev Biophys. .

Abstract

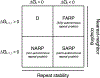
Cooperativity is a hallmark of protein folding, but the thermodynamic origins of cooperativity are difficult to quantify. Tandem repeat proteins provide a unique experimental system to quantify cooperativity due to their internal symmetry and their tolerance of deletion, extension, and in some cases fragmentation into single repeats. Analysis of repeat proteins of different lengths with nearest-neighbor Ising models provides values for repeat folding ([Formula: see text]) and inter-repeat coupling (ΔGi-1,i). In this article, we review the architecture of repeat proteins and classify them in terms of ΔGi and ΔGi-1,i; this classification scheme groups repeat proteins according to their degree of cooperativity. We then present various statistical thermodynamic models, based on the 1D-Ising model, for analysis of different classes of repeat proteins. We use these models to analyze data for highly and moderately cooperative and noncooperative repeat proteins and relate their fitted parameters to overall structural features.

Keywords: Ising model; cooperativity; protein folding; repeat protein; statistical thermodynamics.

PubMed Disclaimer

Figures

Figure 1.
Figure 1.. A thermodynamic classification of linear repeat proteins.
Using the sign of the two Ising energy terms as classifiers, four groups of tandem repeat proteins are generated. Non-autonomous repeat proteins have unstable repeats (ΔGi > 0) but stable interfaces (ΔGi-1,i < 0). Fully-autonomous repeat proteins have stable repeats (ΔGi < 0) but unstable interfaces (ΔGi-1,i > 0). Semi-autonomous repeat proteins have stable repeats and interfaces (ΔGi, ΔGi-1,i < 0). A fourth group, with unstable repeats and interfaces (ΔGi, ΔGi-1,i > 0) would not adopt a folded structure for any number of repeats.
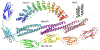
Figure 2.
Figure 2.. Tandem repeat proteins structures.
Ribbon diagrams of a 12-repeat ankyrin array (Michaely et al., 2002), a single repeat from protein A (36), a 3-repeat spectrin array (24), and a six-repeat Ig array from titin (10).
Figure 3.
Figure 3.. Nearest-neighbor model energy terms and statistical weights.
Unfolded and folded repeats are represented by lines and boxes, respectively. The left-hand column shows folding reactions for individual repeats for a two-repeat homopolymer (A; both repeats are labelled R) and a two-repeat heteropolymer (B; repeats are labelled R and X). The equilibrium constant for folding in the context of unfolded neighbors (reactions i and ii) is κR or κX. In the Ising model, the equilibrium constant for folding next to a folded neighbor (reaction iii) is κτ, where τ is the equilibrium constant for interface formation (illustrated by the two vertical transitions). The fractured Ising model permits additional states where adjacent repeats are folded but the interface is not formed (reaction iv). The right-hand column shows statistical weights relative to the reference (unfolded) state.
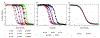
Figure 4.
Figure 4.. Folding transitions of tandem repeat proteins fitted with nearest-neighbor folding models.
Fitted parameters for all data sets are given in Table 4. (A) Consensus ankyrin repeat arrays (a NARP) fitted with a 1D-Ising model. Data are from Aksel et al. (2011). (B) Spectrin repeats R15-R17 (a SARP) fitted with a 1D-ising model modified to include a stabilizing interaction between folded repeat R15 and unfolded repeat R16. Data are from (5). (C) B-domains of Staph. aureus protein A (a FARP) fitted with a fractured Ising model. Data are from (3).
Figure 5.
Figure 5.. Nearest-neighbor free energies of tandem repeat proteins.
Negative values are stabilizing. Naturally occurring and consensus NARPs, SARPs, and FARPs are black, red, and blue, respectively. Rosetta-designed helical repeat proteins (DHRs) are grey. For cANK, values are for the internal (R) repeats. For spectrin, ΔGi and ΔGi-1,i values are from the model that includes a stabilizing interaction between folded repeat 15 and unfolded repeat 16. For BdpA, ΔGi is from the binomial model. For TALEs, ΔGi and ΔGi-1,i values are from Geiger-Schuller & Barrick (2016). For cTPR and 42PR , ΔGi and ΔGi-1,i values are from (20) and (28). For the titin I28e – I32e repeats, ΔGi (and for the I31/I32e pair, ΔGi-1,i) are from Scott et al. (2002). For the four DHR series, ΔGi and ΔGi-1,i values are from Geiger-Schuller et al. (2018). The number line on the right shows average repeat lengths.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

References

    1. Aksel T, Barrick D. 2009. Analysis of repeat-protein folding using nearest-neighbor statistical mechanical models. Meth. Enzymol 455:95–125 - PMC - PubMed
    1. Aksel T, Majumdar A, Barrick D. 2011. The contribution of entropy, enthalpy, and hydrophobic desolvation to cooperativity in repeat-protein folding. Structure. 19(3):349–60 - PMC - PubMed
    1. Arora P, Hammes GG, Oas TG. 2006. Folding Mechanism of a Multiple Independently-Folding Domain Protein: Double B Domain of Protein A†. Biochemistry. 45(40):12312–24 - PubMed
    1. Barrick D 2017. Biomolecular Thermodynamics: From Theory to Application. Boca Raton: CRC Press. 552 pp. 1 edition ed.
    1. Batey S, Clarke J. 2006. Apparent cooperativity in the folding of multidomain proteins depends on the relative rates of folding of the constituent domains. PNAS. 103(48):18113–18 - PMC - PubMed

Publication types

LinkOut - more resources