Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2015 Nov 17;112(46):14248-53.
doi: 10.1073/pnas.1519633112. Epub 2015 Nov 4.

On artifacts in single-molecule force spectroscopy

Pilar Cossio  1 , Gerhard Hummer  1 , Attila Szabo  2
Affiliations

On artifacts in single-molecule force spectroscopy

Pilar Cossio et al. Proc Natl Acad Sci U S A. .

Abstract

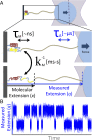
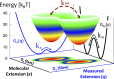
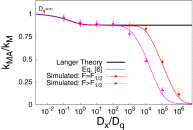
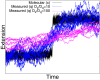
In typical force spectroscopy experiments, a small biomolecule is attached to a soft polymer linker that is pulled with a relatively large bead or cantilever. At constant force, the total extension stochastically changes between two (or more) values, indicating that the biomolecule undergoes transitions between two (or several) conformational states. In this paper, we consider the influence of the dynamics of the linker and mesoscopic pulling device on the force-dependent rate of the conformational transition extracted from the time dependence of the total extension, and the distribution of rupture forces in force-clamp and force-ramp experiments, respectively. For these different experiments, we derive analytic expressions for the observables that account for the mechanical response and dynamics of the pulling device and linker. Possible artifacts arise when the characteristic times of the pulling device and linker become comparable to, or slower than, the lifetimes of the metastable conformational states, and when the highly anharmonic regime of stretched linkers is probed at high forces. We also revisit the problem of relating force-clamp and force-ramp experiments, and identify a linker and loading rate-dependent correction to the rates extracted from the latter. The theory provides a framework for both the design and the quantitative analysis of force spectroscopy experiments by highlighting, and correcting for, factors that complicate their interpretation.

Keywords: anisotropic diffusion; free energy surface; pulling device; unfolding rate.

PubMed Disclaimer

Conflict of interest statement

The authors declare no conflict of interest.

Figures

Fig. 1.
Fig. 1.
(A) Schematic representation of a force spectroscopy experiment. A single molecule (left) is attached to a long linker that is pulled by a large bead trapped in the focus of a laser beam. Stochastic conformational transitions are monitored by measuring the total extension (q). The molecular extension (x) cannot be directly observed. The fluctuations of the molecular extension, τM, are typically fast, on the order of tens of nanoseconds. By contrast, the fluctuations of the apparatus, τA, are slow, on the order of microseconds, but faster than the time between conformational changes of the molecule, kM−1, which goes from milliseconds to seconds. (B) Bistable trajectory of the total extension at constant force, showing infrequent transitions between the folded and unfolded states at small and large q, respectively. The fluctuations of q in the two states are fast compared with the lifetimes of these states.
Fig. 2.
Fig. 2.
Bistable 2D free energy surface G(x,q) as a function of the measured and molecular extensions, q and x, respectively, at the force F1/2 chosen so that the populations are equal. The potentials of mean force along q [GA(q)] and along x [GM(x)] are shown as blue and black curves, respectively. The diffusion is anisotropic, Dx≫Dq, because the latter is essentially the diffusion coefficient of the bead or the tip of an atomic force microscope; kMA is the measured rate (here forward and reverse rates are equal); kM is the molecular rate that one wishes to find; and kA is the rate in the hypothetical case where the system diffuses on the PMF along q with Dq.
Fig. 3.
Fig. 3.
Validation of analytical expressions. Results from 2D anisotropic Brownian dynamics simulations of the measured rate, kMA, normalized by the molecular rate, kM, over a wide range of apparatus diffusion anisotropy, Dx/Dq. The prediction from Langer theory (Eq. 16) is the solid black line. The analytical prediction from Eq. 6 is shown as dashed line, where kA is calculated using the mean first-passage time as in ref. . Error bars represent the SD of the mean. (Inset) Representative transition paths from simulations for the, experimentally inaccessible, molecular extension, x (black), and the measured extension, q, for Dx/Dq=1 (orange) and 10 (blue). See Materials and Methods for details.
Fig. S1.
Fig. S1.
Results from 2D anisotropic Brownian dynamics simulations of the measured unfolding rate, kMA, normalized by the molecular rate, kM, over a wide range of apparatus diffusion anisotropy, Dx/Dq, for two forces, F1/2 (as in Fig. 3) chosen such that the populations of the folded and unfolded states are equal (red) and a larger force F=(3/2)F1/2 (magenta) for which the population of the folded state is smaller than that of the unfolded state. The prediction from Langer theory (Eq. 16) is shown as a solid line. The analytical prediction from Eq. 6 is shown as a dashed line in red for F1/2 and in magenta for F>F1/2, where kA is calculated using the mean first-passage time (36). The simulations at the larger force, F=(3/2)F1/2, were initialized with 5,000 particles distributed according to the Boltzmann distribution in the folded basin (as in ref. 36). Rates are calculated as the inverse of the average lifetime in the folded basin. Error bars represent the SD of the mean. See Materials and Methods for details about the free energy surface and Brownian dynamics.
Fig. S2.
Fig. S2.
Aligned transition paths from 2D anisotropic Brownian dynamics simulations at F1/2. The, experimentally inaccessible, molecular extension, x (black), and the measured extension, q, for Dx/Dq=10 (blue) and 100 (pink). The time range is approximately three times larger than that in Fig. 3, Inset. See Materials and Methods for details about the free energy surface and Brownian dynamics.
Fig. S3.
Fig. S3.
Results from 2D anisotropic Brownian dynamics simulations. Mean transition path time along the measured extension q, normalized by the mean transition path time along the molecular extension x in the limit Dq→∞, 〈tTP(q)〉/〈tTP(x;Dq→∞)〉, over a wide range of apparatus diffusion anisotropy, Dx/Dq. The transition path time was defined as the time to cross over the barrier (determined by the inflection points of the PMF) along the average of 150 aligned transition paths. Error bars represent the SD of the mean, which was calculated using the bootstrap method. The dashed line is a visual guide.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Bell GI. Models for the specific adhesion of cells to cells. Science. 1978;200(4342):618–627. - PubMed
    1. Evans E, Ritchie K. Dynamic strength of molecular adhesion bonds. Biophys J. 1997;72(4):1541–1555. - PMC - PubMed
    1. Hummer G, Szabo A. Kinetics from nonequilibrium single-molecule pulling experiments. Biophys J. 2003;85(1):5–15. - PMC - PubMed
    1. Dudko OK, Filippov AE, Klafter J, Urbakh M. Beyond the conventional description of dynamic force spectroscopy of adhesion bonds. Proc Natl Acad Sci USA. 2003;100(20):11378–11381. - PMC - PubMed
    1. Dudko OK, Hummer G, Szabo A. Intrinsic rates and activation free energies from single-molecule pulling experiments. Phys Rev Lett. 2006;96(10):108101. - PubMed

Publication types

LinkOut - more resources