Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2024 Apr 11;19(4):e0300884.
doi: 10.1371/journal.pone.0300884. eCollection 2024.

Assessing the impact of different contact patterns on disease transmission: Taking COVID-19 as a case

Fenfen Zhang  1   2   3   4   5 , Juan Zhang  3   4   5 , Mingtao Li  6 , Zhen Jin  3   4   5 , Yuqi Wen  7
Affiliations

Assessing the impact of different contact patterns on disease transmission: Taking COVID-19 as a case

Fenfen Zhang et al. PLoS One. .

Abstract

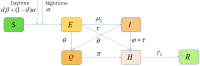
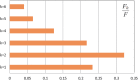
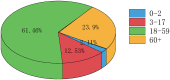
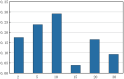
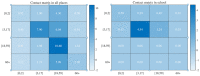
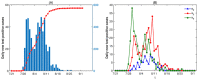
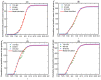
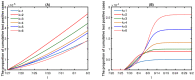
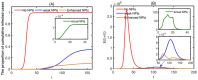
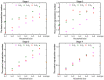
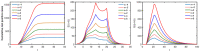
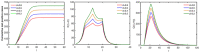
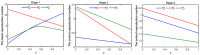
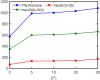
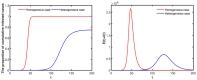
Human-to-human contact plays a leading role in the transmission of infectious diseases, and the contact pattern between individuals has an important influence on the intensity and trend of disease transmission. In this paper, we define regular contacts and random contacts. Then, taking the COVID-19 outbreak in Yangzhou City, China as an example, we consider age heterogeneity, household structure and two contact patterns to establish discrete dynamic models with switching between daytime and nighttime to depict the transmission mechanism of COVID-19 in population. We studied the changes in the reproduction number with different age groups and household sizes at different stages. The effects of the proportion of two contacts patterns on reproduction number were also studied. Furthermore, taking the final size, the peak value of infected individuals in community and the peak value of quarantine infected individuals and nucleic acid test positive individuals as indicators, we evaluate the impact of the number of random contacts, the duration of the free transmission stage and summer vacation on the spread of the disease. The results show that a series of prevention and control measures taken by the Chinese government in response to the epidemic situation are reasonable and effective, and the young and middle-aged adults (aged 18-59) with household size of 6 have the strongest transmission ability. In addition, the results also indicate that increasing the proportion of random contact is beneficial to the control of the infectious disease in the phase with interventions. This work enriches the content of infectious disease modeling and provides theoretical guidance for the prevention and control of follow-up major infectious diseases.

PubMed Disclaimer

Conflict of interest statement

NO authors have competing interests.

Figures

Fig 1
Fig 1. The flowchart of COVID-19 transmission during the free transmission stage.
Fig 2
Fig 2. The flowchart of COVID-19 transmission with intervention measures.
Fig 3
Fig 3. The distribution of household size in Yangzhou City.
Fig 4
Fig 4. Age distribution of population in Yangzhou City.
Fig 5
Fig 5. Distribution of the number of regular contacts in the workplace.
Fig 6
Fig 6. The figures of contact matrix.
The left figure represents the contacts made at all places. The right figure represents the contacts made at school.
Fig 7
Fig 7
(A) The number of daily new test positive cases and cumulative test positive cases for all age groups. (B) The number of daily new test positive cases for age group-ai, i = 2, 3, 4.
Fig 8
Fig 8
(A) The fitting results of age group-a2. (B) The fitting results of age group-a3. (C) The fitting results of age group-a4. (D) The results of the total population.
Fig 9
Fig 9. The proportion of cumulative test positive cases with household size k in the population with the same household size, i.e. IkNk.
The initial values are set as Table 2 and parameter values are set as Table 3.
Fig 10
Fig 10. (A) The proportion of cumulative infected cases of all age groups. (B) The number of infected individuals in society.
The initial values are set as Table 2 and parameter values are set as Table 3. The red line corresponds to the absence of any NPIs. The green line indicates that the NPIs implemented are the same as those in stage 2 (weak NPIs). The green line indicates the actual implementation of NPIs (strong NPIs).
Fig 11
Fig 11. The figures of the basic reproduction number and control reproduction number.
The parameters are set as Table 3.
Fig 12
Fig 12. Effects of the number of random contacts on disease transmission.
x represents the change in the number of random contacts in each age group, positive numbers represent the increase in random contacts, and negative numbers represent the decrease. The other initial values are set as Table 2 and parameter values are set as Table 3.
Fig 13
Fig 13. Effects of the proportion of random contacts on disease transmission.
The initial values are set as Table 2 and parameter values are set as Table 3.
Fig 14
Fig 14. Effects of the proportion of random contacts on the basic reproduction number and control reproduction number.
The parameter values are set as Table 3.
Fig 15
Fig 15. The influence of summer vacation on the spread of COVID-19.
Initial values are set as Table 2 and parameters are set as Table 3. qq=23 and the horizontal axis represents the number of regular contacts between students among age group-a2 at school during daytime.
Fig 16
Fig 16. The impact of heterogeneous and homogeneous mixing and contact patterns on disease transmission without NPIs.
See this image and copyright information in PMC

Similar articles

See all similar articles

References

    1. Holmes KV. SARS-associated coronavirus. New England Journal of Medicine. 2003. May;348(20):1948–1951. doi: 10.1056/NEJMp030078 - DOI - PubMed
    1. Gao H, Yao H, Yang S, et al.. From SARS to MERS: evidence and speculation. Frontiers of medicine. 2016. Dec;10:377–382. doi: 10.1007/s11684-016-0466-7 - DOI - PMC - PubMed
    1. Lai S, Ruktanonchai NW, Zhou L, et al.. Effect of non-pharmaceutical interventions to contain COVID-19 in China. nature. 2020. May;585(7825):410–413. doi: 10.1038/s41586-020-2293-x - DOI - PMC - PubMed
    1. Sarkar K, Khajanchi S, Nieto J J. Modeling and forecasting the COVID-19 pandemic in India. Chaos, Solitons & Fractals. 2020. Oct; 139: 110049. doi: 10.1016/j.chaos.2020.110049 - DOI - PMC - PubMed
    1. Khajanchi S, Sarkar K, Mondal J, et al.. Mathematical modeling of the COVID-19 pandemic with intervention strategies. Results in Physics. 2021. May; 25: 104285. doi: 10.1016/j.rinp.2021.104285 - DOI - PMC - PubMed

Publication types

Supplementary concepts

Grants and funding

This study was financially supported by the National Natural Science Foundation of China in the form of a grant (12231012) received by ZJ. This study was also financially supported by the National Natural Science Foundation of China in the form of a grant (11971278) received by FZ. This study was also financially supported by Shanxi Key Laboratory in the form of a grant (201705D111006) received by ZJ. This study was also financially supported by Key R&D projects of Shanxi Province in the form of a grant (202003D31011/GZ) received by ZJ. This study was also financially supported by Shanxi Provincial Research and Development Program in the form of a grant (202102130501002) received by ZJ. This study was also financially supported by Health Commission of Shanxi Province in the form of a grant (2020XM18) received by ZJ. This study was also financially supported by Selected funding Project for Scientific and technological activities of overseas students in 2021 in the form of a grant (20210009) received by JZ. This study was also financially supported by Fundamental Research Program of Shanxi Province in the form of a grant (20210302124608) received by FZ. This study was also financially supported by Fundamental Research Program of Shanxi Province in the form of a grant (202103021224095) received by LT. This study was also financially supported by Research of Technological Important Programs in the city of Lvliang, China in the form of a grant (2022GXYF18) received by ZJ. The funders had no role in study design, data collection and analysis, decision to publish, or preparation of the manuscript.