Microtubule Simulations Provide Insight into the Molecular Mechanism Underlying Dynamic Instability
- PMID: 32413312
- PMCID: PMC7300308
- DOI: 10.1016/j.bpj.2020.04.028
Microtubule Simulations Provide Insight into the Molecular Mechanism Underlying Dynamic Instability
Abstract
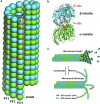
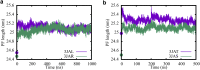
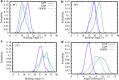
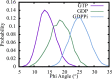
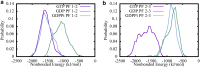
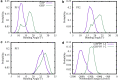
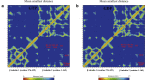
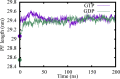
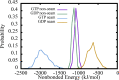
The dynamic instability of microtubules (MTs), which refers to their ability to switch between polymerization and depolymerization states, is crucial for their function. It has been proposed that the growing MT ends are protected by a "GTP cap" that consists of GTP-bound tubulin dimers. When the speed of GTP hydrolysis is faster than dimer recruitment, the loss of this GTP cap will lead the MT to undergo rapid disassembly. However, the underlying atomistic mechanistic details of the dynamic instability remains unclear. In this study, we have performed long-time atomistic molecular dynamics simulations (1 μs for each system) for MT patches as well as a short segment of a closed MT in both GTP- and GDP-bound states. Our results confirmed that MTs in the GDP state generally have weaker lateral interactions between neighboring protofilaments (PFs) and less cooperative outward bending conformational change, where the difference between bending angles of neighboring PFs tends to be larger compared with GTP ones. As a result, when the GDP state tubulin dimer is exposed at the growing MT end, these factors will be more likely to cause the MT to undergo rapid disassembly. We also compared simulation results between the special MT seam region and the remaining material and found that the lateral interactions between MT PFs at the seam region were comparatively much weaker. This finding is consistent with the experimental suggestion that the seam region tends to separate during the disassembly process of an MT.
Copyright © 2020 Biophysical Society. Published by Elsevier Inc. All rights reserved.
Figures
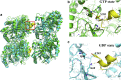


Similar articles
-
Structural transitions in the GTP cap visualized by cryo-electron microscopy of catalytically inactive microtubules.Proc Natl Acad Sci U S A. 2022 Jan 11;119(2):e2114994119. doi: 10.1073/pnas.2114994119. Proc Natl Acad Sci U S A. 2022. PMID: 34996871 Free PMC article.
-
Intrinsic bending of microtubule protofilaments.Structure. 2011 Mar 9;19(3):409-17. doi: 10.1016/j.str.2010.12.020. Structure. 2011. PMID: 21397191
-
Intrinsic bending and structural rearrangement of tubulin dimer: molecular dynamics simulations and coarse-grained analysis.Biophys J. 2008 Sep;95(5):2487-99. doi: 10.1529/biophysj.108.129072. Epub 2008 May 30. Biophys J. 2008. PMID: 18515385 Free PMC article.
-
How tubulin subunits are lost from the shortening ends of microtubules.J Struct Biol. 1997 Mar;118(2):107-18. doi: 10.1006/jsbi.1997.3844. J Struct Biol. 1997. PMID: 9126637 Review.
-
Guanosine-5'-triphosphate hydrolysis and tubulin polymerization. Review article.Mol Cell Biochem. 1982 Sep 3;47(2):97-113. doi: 10.1007/BF00234410. Mol Cell Biochem. 1982. PMID: 6755216 Review.
Cited by
-
Equilibrium mechanisms of self-limiting assembly.Rev Mod Phys. 2021 Apr-Jun;93(2):025008. doi: 10.1103/revmodphys.93.025008. Epub 2021 Jun 11. Rev Mod Phys. 2021. PMID: 35221384 Free PMC article.
-
Polymorphic self-assembly of helical tubules is kinetically controlled.Soft Matter. 2022 Sep 14;18(35):6716-6728. doi: 10.1039/d2sm00679k. Soft Matter. 2022. PMID: 36039801 Free PMC article.
-
Microtubule instability driven by longitudinal and lateral strain propagation.PLoS Comput Biol. 2020 Sep 2;16(9):e1008132. doi: 10.1371/journal.pcbi.1008132. eCollection 2020 Sep. PLoS Comput Biol. 2020. PMID: 32877399 Free PMC article.
-
Structural transitions in the GTP cap visualized by cryo-electron microscopy of catalytically inactive microtubules.Proc Natl Acad Sci U S A. 2022 Jan 11;119(2):e2114994119. doi: 10.1073/pnas.2114994119. Proc Natl Acad Sci U S A. 2022. PMID: 34996871 Free PMC article.
-
Unveiling the catalytic mechanism of GTP hydrolysis in microtubules.Proc Natl Acad Sci U S A. 2023 Jul 4;120(27):e2305899120. doi: 10.1073/pnas.2305899120. Epub 2023 Jun 26. Proc Natl Acad Sci U S A. 2023. PMID: 37364095 Free PMC article.
References
-
- Hyams J.S., Lloyd C.W. Microtubules. In: Harford J.B., editor. Modern Cell Biology. Wiley-Liss; 1993. pp. 1–439.
-
- Nogales E., Whittaker M., Downing K.H. High-resolution model of the microtubule. Cell. 1999;96:79–88. - PubMed
-
- Amos L., Klug A. Arrangement of subunits in flagellar microtubules. J. Cell Sci. 1974;14:523–549. - PubMed
-
- Mitchison T., Kirschner M. Dynamic instability of microtubule growth. Nature. 1984;312:237–242. - PubMed
Publication types
MeSH terms
Substances
Grants and funding
LinkOut - more resources
Full Text Sources
Research Materials
Miscellaneous

