Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2019 Sep 27;16(158):20190334.
doi: 10.1098/rsif.2019.0334. Epub 2019 Sep 4.

Hepatitis C virus modelled as an indirectly transmitted infection highlights the centrality of injection drug equipment in disease dynamics

Miles D Miller-Dickson  1 , Victor A Meszaros  1 , Salvador Almagro-Moreno  2   3 , C Brandon Ogbunugafor  1
Affiliations

Hepatitis C virus modelled as an indirectly transmitted infection highlights the centrality of injection drug equipment in disease dynamics

Miles D Miller-Dickson et al. J R Soc Interface. .

Abstract

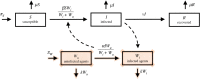
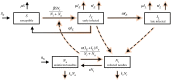
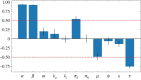
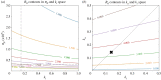
The hepatitis C virus (HCV) epidemic often occurs through the persistence of injection drug use. Mathematical models have been useful in understanding various aspects of the HCV epidemic, and especially, the importance of new treatment measures. Until now, however, few models have attempted to understand HCV in terms of an interaction between the various actors in an HCV outbreak-hosts, viruses and the needle injection equipment. In this study, we apply perspectives from the ecology of infectious diseases to model the transmission of HCV among a population of injection drug users. The products of our model suggest that modelling HCV as an indirectly transmitted infection-where the injection equipment serves as an environmental reservoir for infection-facilitates a more nuanced understanding of disease dynamics, by animating the underappreciated actors and interactions that frame disease. This lens may allow us to understand how certain public health interventions (e.g. needle exchange programmes) influence HCV epidemics. Lastly, we argue that this model is of particular importance in the light of the modern opioid epidemic, which has already been associated with outbreaks of viral diseases.

Keywords: ecology of infectious disease; epidemiology; mathematical modelling.

PubMed Disclaimer

Conflict of interest statement

We declare we have no competing interests.

Figures

Figure 1.
Figure 1.
Adapted SIR compartmental diagram. This depicts a standard SIR style compartmental model with the added compartments (shaded) corresponding to the W.A.I.T. environment. Note the dynamical properties of the Wi and Wu compartments. (Online version in colour.)
Figure 2.
Figure 2.
HCV compartmental diagram. Red arrows highlight flow of disease through the system, and where there is a colour/transparency gradient there is a flow of infection away from an infected compartment towards an uninfected one. (Online version in colour.)
Figure 3.
Figure 3.
R0 sensitivity in HCV: the partial rank correlation coefficient (PRCC). A PRCC calculation was performed for R0 using Latin hypercube sampling. Parameters were sampled from uniform distributions with widths specified by the ranges given in table 1. The PRCC calculation was repeated for 50 independent iterations. The averages of these iterations are shown here, with the standard deviations for each parameter shown as the error bars. (Online version in colour.)
Figure 4.
Figure 4.
HCV R0 as a function of various model features. (a) The relationship between the rate of acquisition of clean needles πN and the discard rate of infected needles ki with respect to various values of R0. The curves are contours of R0 and are labelled by the associated R0 value. The vertical and horizontal dashed lines indicate the chosen values for their respective parameters (we fix ku to the value specified in table 1). (b) The relationship between the infected and uninfected needle discard rate, with respect to R0. The diagonal line represents where ku = ki. The ‘x’ indicates the value chosen for ku and ki in the model (we set ku = ki in the model). Notice that moving upwards along this diagonal increases R0. (Online version in colour.)
Figure 5.
Figure 5.
The dynamics of susceptible (blue), early-infected (orange) and late-infected (green) populations in two parameter regimes: high and low ϵ, the conversion rate of needles from infected to uninfected. The solid lines represent the dynamics for ϵ=2 day−1 (high ϵ), and dashed lines are the dynamics for ϵ=0.33 d−1 (low ϵ). In the high-ϵ regime, we find that the susceptible population at equilibrium is ≈4 times that of the low-ε regime, and the infected populations are each ≈89% of their low-ϵ counterparts at equilibrium (note the log scale on the y-axis). (Online version in colour.)
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

References

    1. Dick EC, Jennings LC, Mink KA, Wartgow CD, Inborn SL. 1987. Aerosol transmission of rhinovirus colds. J. Infect. Dis. 156, 442–448. (10.1093/infdis/156.3.442) - DOI - PubMed
    1. Abad FX, Pinto RM, Bosch A. 1994. Survival of enteric viruses on environmental fomites. Appl. Environ. Microbiol. 60, 3704–3710. - PMC - PubMed
    1. Codeço CT. 2001. Endemic and epidemic dynamics of cholera: the role of the aquatic reservoir. BMC Infect. Dis. 1, 1 (10.1186/1471-2334-1-1) - DOI - PMC - PubMed
    1. Boone SA, Gerba CP. 2007. Significance of fomites in the spread of respiratory and enteric viral disease. Appl. Environ. Microbiol. 73, 1687–1696. (10.1128/AEM.02051-06) - DOI - PMC - PubMed
    1. Weber TP, Stilianakis NI. 2008. Inactivation of influenza a viruses in the environment and modes of transmission: a critical review. J. Infect. 57, 361–373. (10.1016/j.jinf.2008.08.013) - DOI - PMC - PubMed

Publication types