Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2018 Jun 1;46(10):4883-4892.
doi: 10.1093/nar/gky270.

Improving RNA nearest neighbor parameters for helices by going beyond the two-state model

Aleksandar Spasic  1   2 , Kyle D Berger  1   2 , Jonathan L Chen  2   3 , Matthew G Seetin  1   2 , Douglas H Turner  2   3 , David H Mathews  1   2   4
Affiliations

Improving RNA nearest neighbor parameters for helices by going beyond the two-state model

Aleksandar Spasic et al. Nucleic Acids Res. .

Abstract

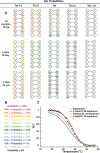
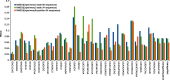
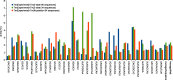
RNA folding free energy change nearest neighbor parameters are widely used to predict folding stabilities of secondary structures. They were determined by linear regression to datasets of optical melting experiments on small model systems. Traditionally, the optical melting experiments are analyzed assuming a two-state model, i.e. a structure is either complete or denatured. Experimental evidence, however, shows that structures exist in an ensemble of conformations. Partition functions calculated with existing nearest neighbor parameters predict that secondary structures can be partially denatured, which also directly conflicts with the two-state model. Here, a new approach for determining RNA nearest neighbor parameters is presented. Available optical melting data for 34 Watson-Crick helices were fit directly to a partition function model that allows an ensemble of conformations. Fitting parameters were the enthalpy and entropy changes for helix initiation, terminal AU pairs, stacks of Watson-Crick pairs and disordered internal loops. The resulting set of nearest neighbor parameters shows a 38.5% improvement in the sum of residuals in fitting the experimental melting curves compared to the current literature set.

PubMed Disclaimer

Figures

Figure 1.
Figure 1.
Comparison of melting for duplex 5′-AUCGGUA/3′-UAGCCAU with total strand concentration of 6 μM. Panel A provides pair probabilities at the experimental melting temperature (Tm = 37.1°C), Tm ±5°C and Tm ±10°C using the literature two-state-derived parameters (16) and the newly fitted parameters. Panel B is the pair probability color annotation key. In panel C, the estimated melting curves are compared to the fraction of maximal base pairs as a function of temperature derived from the optical melting curves (see Materials and Methods). Diamonds are the experimental melting data. The red line is the prediction using the newly fitted parameters, the blue line is the prediction using the literature two-state-derived nearest neighbor parameters (16) and the green line is the prediction using the parameters from the two-state fit on 34 duplexes available in this work. All melting curves were computed using a partition function. Melting temperatures for the experiment, the partition function parameters, literature two-state parameters, and two-state parameters with 34 duplexes are 37.1, 38.0, 33.7 and 35.9°C, respectively. RMSDs between experimental and melting curves from two-state fit to 90 duplexes (blue line), partition function fit to 34 duplexes (red line) and two-state fit to 34 duplexes (green line) were 0.12, 0.03 and 0.06 respectively.
Figure 2.
Figure 2.
Comparison of root mean square deviations (RMSD) of fraction of bases paired between experimental and estimated optical melting curves. Three sets of parameters were compared: the literature two-state parameters (blue bars), the parameters from two-state fit performed with 34 duplexes (green) and the fit with the partition function (red). The predictions of two sets of parameters derived here (green and red bars) were derived using the jackknife method. The final two columns are comparisons of the averages per unique duplex and averages per melt, as each duplex had multiple melts. Note that the RMSD is calculated for fraction of bases paired, which is bounded between 0 and 1, so the improvement in the fits is substantial. Sequences are written with 5′ end at top.
Figure 3.
Figure 3.
Comparison of absolute values of differences between predicted melting temperatures and measured melting temperatures. Blue bars are the differences between melting temperatures calculated directly from melting experiments and predicted using the literature two-state model derived nearest neighbor parameters (16). Green bars are the differences between experimental melting temperatures and melting temperatures predicted using the two-state model and the 34 duplexes used in this work. Red bars are differences between experimental melting temperatures and melting temperatures calculated using the parameters derived in this work by fitting to the partition function. The predictions of two sets of parameters derived here (green and red bars) were derived using the jackknife method. The last two bars are averages over the 34 unique duplexes and over all individual melts, as each duplex had multiple melts. Sequences are written with 5′ end at top.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Turner D.H., Mathews D.H.. NNDB: the nearest neighbor parameter database for predicting stability of nucleic acid secondary structure. Nucleic Acids Res. 2010; 38:D280–D282. - PMC - PubMed
    1. Reuter J.S., Mathews D.H.. RNAstructure: software for RNA secondary structure prediction and analysis. BMC Bioinformatics. 2010; 11:129. - PMC - PubMed
    1. Lorenz R., Bernhart S.H., Siederdissen C.H. zu, Tafer H., Flamm C., Stadler P.F., Hofacker I.L.. ViennaRNA Package 2.0. Algorithms Mol. Biol. 2011; 6:26. - PMC - PubMed
    1. Zuker M. Mfold web server for nucleic acid folding and hybridization prediction. Nucleic Acids Res. 2003; 31:3406–3415. - PMC - PubMed
    1. Markham N.R., Zuker M.. Keith JM. UNAFold. Bioinformatics. 2008; 453:Totowa: Humana Press; 3–31.

Publication types