Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2018 Mar 5:7:e32354.
doi: 10.7554/eLife.32354.

Mechanochemical coupling and bi-phasic force-velocity dependence in the ultra-fast ring ATPase SpoIIIE

Ninning Liu #  1   2 , Gheorghe Chistol #  1   3 , Yuanbo Cui  1   4 , Carlos Bustamante  1   2   3   4   5   6   7
Affiliations

Mechanochemical coupling and bi-phasic force-velocity dependence in the ultra-fast ring ATPase SpoIIIE

Ninning Liu et al. Elife. .

Abstract

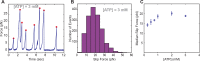
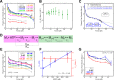
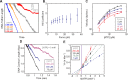
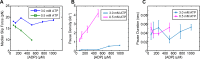
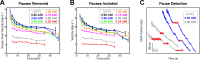
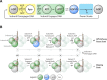
Multi-subunit ring-shaped ATPases are molecular motors that harness chemical free energy to perform vital mechanical tasks such as polypeptide translocation, DNA unwinding, and chromosome segregation. Previously we reported the intersubunit coordination and stepping behavior of the hexameric ring-shaped ATPase SpoIIIE (Liu et al., 2015). Here we use optical tweezers to characterize the motor's mechanochemistry. Analysis of the motor response to external force at various nucleotide concentrations identifies phosphate release as the likely force-generating step. Analysis of SpoIIIE pausing indicates that pauses are off-pathway events. Characterization of SpoIIIE slipping behavior reveals that individual motor subunits engage DNA upon ATP binding. Furthermore, we find that SpoIIIE's velocity exhibits an intriguing bi-phasic dependence on force. We hypothesize that this behavior is an adaptation of ultra-fast motors tasked with translocating DNA from which they must also remove DNA-bound protein roadblocks. Based on these results, we formulate a comprehensive mechanochemical model for SpoIIIE.

Keywords: B. subtilis; Ftsk/SpoIIIE; Ring ATPase; biochemistry; chemical biology; mechanochemistry; molecular biophysics; optical tweezers; single-molecule; structural biology.

PubMed Disclaimer

Conflict of interest statement

NL, GC, YC, CB No competing interests declared

Figures

Figure 1.
Figure 1.. Optical tweezer experimental geometry in constant force and passive mode.
(a) Optical tweezer geometry. (b) Representative single-molecule traces of SpoIIIE translocation in passive mode. The trap position is fixed and as SpoIIIE pulls the bead out of the trap, the force on the trapped bead increases. (c) Representative single-molecule traces of SpoIIIE translocation in constant force mode. The optical trap position is continuously adjusted to maintain a constant force on the trapped bead. (d) Comparison of pause-free velocity measured in constant force mode and passive mode at [ATP]=3 mM. Error-bars represent the standard error of the mean (SEM). (e) Trace displaying a slip in constant force mode. (f) Slip density at different opposing force and [ATP]. Error bars represent the square root of the number of events.
Figure 1—figure supplement 1.
Figure 1—figure supplement 1.. Slipping behavior of SpoIIIE.
(a) Single-molecule trace of SpoIIIE translocation acquired in passive mode. Red dots indicate force where a slip was detected. (b) Histogram of pull forces. (c) Median pull force of SpoIIIE across various ATP conditions. Error bars display the standard error estimated from bootstrapping.
Figure 2.
Figure 2.. Force-velocity dependence displayed of SpoIIIE.
(a) Pause-free translocation velocity versus opposing force at various [ATP], 5 µM ADP, and 5 µM Pi. Error-bars represent the SEM. (b) Hill coefficient derived from fitting translocation velocity versus [ATP] at various opposing forces. Error bars represent the standard error of the fit (SEF). (c) Pause-free velocity versus opposing force compiled from data at 5, 3, and 2 mM ATP. Error-bars represent the SEM. Gray and blue curves represent fits to the two different models depicted in the inset. Analytic expressions and fit parameters for the models are given in Figure 2—figure supplement 2. (d) Generalized kinetic cycle for an ATPase subunit. The first block consists of all rate constants k±1, k±2, … up to the first irreversible transition kj (purple). The second block comprises the remaining rate constants (green). (e) Pause-free velocity versus opposing force at various [ADP] and 3 mM ATP. (f) Vmax and KM values as a function of [ADP] at low opposing force (5 pN). Solid lines are fits to a competitive inhibition model, Ki = 129 ± 19 μM. Error-bars represent the SEF. (g) Pause-free velocity versus opposing force under high [Pi] conditions and 3 mM ATP. Error bars represent the SEM.
Figure 2—figure supplement 1.
Figure 2—figure supplement 1.. Michaelis-Menten fits to SpoIIIE translocation.
(a) Passive mode traces of SpoIIIE translocation across various [ATP]. (b) Hill coefficient derived from fitting translocation velocity versus [ATP] at various opposing forces. Error bars represent the standard error of the fit (SEF). (c) Examples of Michaelis-Menten fits to translocation data at different opposing forces. (d) Representative translocation traces acquired in passive mode at 3 mM ATP and various ADP concentrations. Error-bars represent the SEM. (e) Lineweaver-Burke plots at various [ADP] (v denotes the pause-free velocity). Dotted lines represent the Michaelis-Menten fits. The solid purple line marks the y-intercept. Error bars represent the SEM.
Figure 2—figure supplement 2.
Figure 2—figure supplement 2.. Diagrams of mechanochemical models with two force-dependent transitions.
(a) Diagram, analytical expression, and fit parameters for the linear model. Analytical expression for the force-velocity dependence, and parameters derived from fitting this expression to the consolidated force-velocity curve depicted in Figure 2C. (b) Diagram illustrating the branched model.
Figure 2—figure supplement 3.
Figure 2—figure supplement 3.. SpoIIIE slipping behavior in presence of ADP.
(a) Median slip force of SpoIIIE as a function of [ADP] at high (3 mM) and low (0.5 mM) [ATP]. Error bars represent the SEM. (b) Pause density versus [ADP] at both high and low ATP concentrations. Error bars display the square root of the pause number. (c) Mean pause durations calculated from single-exponential fits versus ADP concentration at high and low [ATP]. Error bars represent the 95% CI of the fit.
Figure 2—figure supplement 4.
Figure 2—figure supplement 4.. SpoIIIE pause detection and pause removal.
(a) Pause-free translocation velocity versus opposing force at various [ATP], reproduced from figure panel 2A. (b) Translocation velocity versus opposing force. Pauses were not removed for velocity calculations. (c) Examples of pauses scored by the pause detection algorithm (red) in data collected in passive mode at two ATP conditions.
Figure 3.
Figure 3.. Characterization of spontaneous pausing by SpoIIIE.
(a) Examples of SpoIIIE translocation trajectories acquired at low force (5 pN) and various ATP concentrations with detected pauses highlighted in red. (b) Measured pause density (solid lines) and corrected pause density (dashed lines) accounting for the missed pauses versus pause-free velocity at 5 pN. (c) Measured and corrected pause densities versus ATP concentration at 5 pN. (d) The mean pause lifetime calculated by fitting the distribution of pause durations to a single exponential (see inset). Error-bars represent the SEF. (Inset) Distribution of pause durations at 250 μM [ATP] (green) fit to a single-exponential decay (dashed line). The mean pause lifetime estimates at high [ATP] are less accurate due to the low number of detectable pauses. (e) Measured pause density versus opposing force at various [ATP]. (f) Mean pause lifetimes versus opposing force at the two lowest [ATP], where the number of pauses was sufficiently high to accurately estimate the lifetimes from fits. Error bars from fits represent 95% CI from fits. Error bars of pause density estimated from square root of the number of pause events.
Figure 4.
Figure 4.. SpoIIIE mechanochemistry model.
(a) Mechanochemical cycle for a single SpoIIIE subunit. (b) Mechanochemical cycle for the entire SpoIIIE homo-hexamer.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Baumann CG, Smith SB, Bloomfield VA, Bustamante C. Ionic effects on the elasticity of single DNA molecules. PNAS. 1997;94:6185–6190. doi: 10.1073/pnas.94.12.6185. - DOI - PMC - PubMed
    1. Berndsen ZT, Keller N, Smith DE. Continuous allosteric regulation of a viral packaging motor by a sensor that detects the density and conformation of packaged DNA. Biophysical Journal. 2015;108:315–324. doi: 10.1016/j.bpj.2014.11.3469. - DOI - PMC - PubMed
    1. Burton BM, Marquis KA, Sullivan NL, Rapoport TA, Rudner DZ. The ATPase SpoIIIE transports DNA across fused septal membranes during sporulation in Bacillus subtilis. Cell. 2007;131:1301–1312. doi: 10.1016/j.cell.2007.11.009. - DOI - PMC - PubMed
    1. Bustamante C, Chemla YR, Forde NR, Izhaky D. Mechanical processes in biochemistry. Annual Review of Biochemistry. 2004;73:705–748. doi: 10.1146/annurev.biochem.72.121801.161542. - DOI - PubMed
    1. Chemla YR, Aathavan K, Michaelis J, Grimes S, Jardine PJ, Anderson DL, Bustamante C. Mechanism of force generation of a viral DNA packaging motor. Cell. 2005;122:683–692. doi: 10.1016/j.cell.2005.06.024. - DOI - PubMed

Publication types

MeSH terms

LinkOut - more resources