Existence of and decay to equilibrium of the filament end density along the leading edge of the lamellipodium

doi:10.1007/s00285-016-1027-z

. 2017 Jan;74(1-2):169-193.

doi: 10.1007/s00285-016-1027-z. Epub 2016 May 20.

Existence of and decay to equilibrium of the filament end density along the leading edge of the lamellipodium

Angelika Manhart^{1

2}, Christian Schmeiser³

Affiliations

¹ CIMS, New York University, 251 Mercer Street, New York, NY, 10012, USA. angelika.manhart@cims.nyu.edu.
² Faculty of Mathematics, University of Vienna, Oskar-Morgenstern Platz 1, 1090, Vienna, Austria. angelika.manhart@cims.nyu.edu.
³ Faculty of Mathematics, University of Vienna, Oskar-Morgenstern Platz 1, 1090, Vienna, Austria.

PMID: 27206776
PMCID: PMC5206285
DOI: 10.1007/s00285-016-1027-z

Existence of and decay to equilibrium of the filament end density along the leading edge of the lamellipodium

Angelika Manhart et al. J Math Biol. 2017 Jan.

. 2017 Jan;74(1-2):169-193.

doi: 10.1007/s00285-016-1027-z. Epub 2016 May 20.

Authors

Angelika Manhart^{1

2}, Christian Schmeiser³

Affiliations

¹ CIMS, New York University, 251 Mercer Street, New York, NY, 10012, USA. angelika.manhart@cims.nyu.edu.
² Faculty of Mathematics, University of Vienna, Oskar-Morgenstern Platz 1, 1090, Vienna, Austria. angelika.manhart@cims.nyu.edu.
³ Faculty of Mathematics, University of Vienna, Oskar-Morgenstern Platz 1, 1090, Vienna, Austria.

PMID: 27206776
PMCID: PMC5206285
DOI: 10.1007/s00285-016-1027-z

Abstract

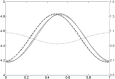
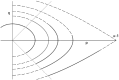
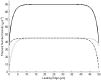
A model for the dynamics of actin filament ends along the leading edge of the lamellipodium is analyzed. It contains accounts of nucleation by branching, of deactivation by capping, and of lateral flow along the leading edge by polymerization. A nonlinearity arises from a Michaelis-Menten type modeling of the branching process. For branching rates large enough compared to capping rates, the existence and stability of nontrivial steady states is investigated. The main result is exponential convergence to nontrivial steady states, proven by investigating the decay of an appropriate Lyapunov functional.

Keywords: Actin; Lamellipodium; Lyapunov function.

PubMed Disclaimer

Figures

**Fig. 1**
Lateral flow. *Solid* and *dashed lines* represent the present and, respectively, a future state of filaments of the leading edge (drawing courtesy of J. Vic Small)

**Fig. 2**
Illustration of the proof of Proposition 2

**Fig. 3**
Approximate and numerical solution for $α = 10$ and $ε = 0.1$ with c(x) given by (26). The end density of the *right* moving filaments u is depicted in *dark* and *light blue* (*dashed*) and that of the *left* moving filaments v (*solid*) in *red* and *orange*. *Thin lines* (*red* and *dark blue*) are the asymptotic approximations, *thick lines* (*orange* and *light blue*) are the numerical solution of the time dependent problem after $t = 20$ and the left y-axis applies. In *green* (*dotted*) the lateral flow c(x) is depicted, the values are in relation to the right y-axis

**Fig. 4**
This figure shows the trajectories in (p, q)-phase space of the stationary equation with constant c and (*DBC*). The *orange*, *diagonal lines* represent the boundary conditions $q = - p$ at $x = 0$ and $q = p$ at $x = 1$ . *Dashed curves* represent (29) for different values of $E_{0}$ and the *blue lines* the corresponding solutions curves fulfilling $p (0) + q (0) = 0$ . The *red line* is desired the solution satisfying both boundary conditions

**Fig. 5**
Density distribution of filaments along the leading edge for Dirichlet boundary conditions as given in Eq. (2) using the equation prior to non-dimensionalization. *Red-dotted* and *blue-dashed lines* refer to *right* and *left* moving filaments respectively; the *black-solid line* shows the total filament end density. Parameters (not scaled): $L = 50 μ m$ , $κ_{br} = 10 / s$ , $κ_{cap} = 0.1 / s$ , $c_{rec} = 9.1 / (μ m s)$ , $c (x) \equiv 0.305 μ m / s$

See this image and copyright information in PMC

Cited by

Computational estimates of mechanical constraints on cell migration through the extracellular matrix.
Maxian O, Mogilner A, Strychalski W. Maxian O, et al. PLoS Comput Biol. 2020 Aug 27;16(8):e1008160. doi: 10.1371/journal.pcbi.1008160. eCollection 2020 Aug. PLoS Comput Biol. 2020. PMID: 32853248 Free PMC article.

References

1. Atilgan E, Wirtz D, Sun SX. Morphology of the lamellipodium and organization of actin filaments at the leading edge of crawling cells. Biophys J. 2005;89:3589–3602. doi: 10.1529/biophysj.105.065383. - DOI - PMC - PubMed
1. Blanchoin L, Amann K, Higgs H, Marchand JB, Kaiser DA, Pollard TD. Direct observation of dendritic actin filament networks nucleated by Arp2/3 complex and WASP/Scar proteins. Nature. 2000;404(6781):1007–1011. doi: 10.1038/35010008. - DOI - PubMed
1. Crandall MG, Rabinowitz PH. Bifurcation from simple eigenvalues. J Funct Anal. 1971;8(2):321–340. doi: 10.1016/0022-1236(71)90015-2. - DOI
1. Grimm HP, Verkhovsky AB, Mogilner A, Meister J-J. Analysis of actin dynamics at the leading edge of crawling cells: implications for the shape of keratocyte lamellipodia. Eur Biophys J. 2003;32(6):563–577. doi: 10.1007/s00249-003-0300-4. - DOI - PubMed
1. Hui KL, Wang C, Grooman B, Wayt J, Upadhyaya A. Membrane dynamics correlate with formation of signaling clusters during cell spreading. Biophys J. 2012;102(7):1524–1533. doi: 10.1016/j.bpj.2012.02.015. - DOI - PMC - PubMed

MeSH terms

Actions
Actions
Actions
Actions

LinkOut - more resources

Full Text Sources
Other Literature Sources
- scite Smart Citations

[1] Atilgan E, Wirtz D, Sun SX. Morphology of the lamellipodium and organization of actin filaments at the leading edge of crawling cells. Biophys J. 2005;89:3589–3602. doi: 10.1529/biophysj.105.065383. - DOI - PMC - PubMed

[2] Atilgan E, Wirtz D, Sun SX. Morphology of the lamellipodium and organization of actin filaments at the leading edge of crawling cells. Biophys J. 2005;89:3589–3602. doi: 10.1529/biophysj.105.065383. - DOI - PMC - PubMed

[3] Blanchoin L, Amann K, Higgs H, Marchand JB, Kaiser DA, Pollard TD. Direct observation of dendritic actin filament networks nucleated by Arp2/3 complex and WASP/Scar proteins. Nature. 2000;404(6781):1007–1011. doi: 10.1038/35010008. - DOI - PubMed

[4] Blanchoin L, Amann K, Higgs H, Marchand JB, Kaiser DA, Pollard TD. Direct observation of dendritic actin filament networks nucleated by Arp2/3 complex and WASP/Scar proteins. Nature. 2000;404(6781):1007–1011. doi: 10.1038/35010008. - DOI - PubMed

[5] Crandall MG, Rabinowitz PH. Bifurcation from simple eigenvalues. J Funct Anal. 1971;8(2):321–340. doi: 10.1016/0022-1236(71)90015-2. - DOI

[6] Crandall MG, Rabinowitz PH. Bifurcation from simple eigenvalues. J Funct Anal. 1971;8(2):321–340. doi: 10.1016/0022-1236(71)90015-2. - DOI

[7] Grimm HP, Verkhovsky AB, Mogilner A, Meister J-J. Analysis of actin dynamics at the leading edge of crawling cells: implications for the shape of keratocyte lamellipodia. Eur Biophys J. 2003;32(6):563–577. doi: 10.1007/s00249-003-0300-4. - DOI - PubMed

[8] Grimm HP, Verkhovsky AB, Mogilner A, Meister J-J. Analysis of actin dynamics at the leading edge of crawling cells: implications for the shape of keratocyte lamellipodia. Eur Biophys J. 2003;32(6):563–577. doi: 10.1007/s00249-003-0300-4. - DOI - PubMed

[9] Hui KL, Wang C, Grooman B, Wayt J, Upadhyaya A. Membrane dynamics correlate with formation of signaling clusters during cell spreading. Biophys J. 2012;102(7):1524–1533. doi: 10.1016/j.bpj.2012.02.015. - DOI - PMC - PubMed

[10] Hui KL, Wang C, Grooman B, Wayt J, Upadhyaya A. Membrane dynamics correlate with formation of signaling clusters during cell spreading. Biophys J. 2012;102(7):1524–1533. doi: 10.1016/j.bpj.2012.02.015. - DOI - PMC - PubMed

Save citation to file

Email citation

Add to Collections

Add to My Bibliography

Your saved search

Create a file for external citation management software

Your RSS Feed

Existence of and decay to equilibrium of the filament end density along the leading edge of the lamellipodium

Affiliations

Existence of and decay to equilibrium of the filament end density along the leading edge of the lamellipodium

Authors

Affiliations

Abstract

Figures

Similar articles

Cited by

References

MeSH terms

LinkOut - more resources

Full Text Sources

Other Literature Sources