Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2015 Jan 20;10(1):e0115187.
doi: 10.1371/journal.pone.0115187. eCollection 2015.

Abortive and propagating intracellular calcium waves: analysis from a hybrid model

Nara Guisoni  1 , Paola Ferrero  2 , Carla Layana  3 , Luis Diambra  3
Affiliations

Abortive and propagating intracellular calcium waves: analysis from a hybrid model

Nara Guisoni et al. PLoS One. .

Abstract

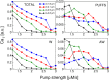
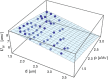
The functional properties of inositol(1,4,5)-triphosphate (IP3) receptors allow a variety of intracellular Ca(2+) phenomena. In this way, global phenomena, such as propagating and abortive Ca(2+) waves, as well as local events such as puffs, have been observed. Several experimental studies suggest that many features of global phenomena (e.g., frequency, amplitude, speed wave) depend on the interplay of biophysical processes such as diffusion, buffering, efflux and influx rates, which in turn depend on parameters such as buffer concentration, Ca(2+) pump density, cytosolic IP3 level, and intercluster distance. Besides, it is known that cells are able to modify some of these parameters in order to regulate the Ca(2+) signaling. By using a hybrid model, we analyzed different features of the hierarchy of calcium events as a function of two relevant parameters for the calcium signaling, the intercluster distance and the pump strength or intensity. In the space spanned by these two parameters, we found two modes of calcium dynamics, one dominated by abortive calcium waves and the other by propagating waves. Smaller distances between the release sites promote propagating calcium waves, while the increase of the efflux rate makes the transition from propagating to abortive waves occur at lower values of intercluster distance. We determined the frontier between these two modes, in the parameter space defined by the intercluster distance and the pump strength. Furthermore, we found that the velocity of simulated calcium waves accomplishes Luther's law, and that an effective rate constant for autocatalytic calcium production decays linearly with both the intercluster distance and the pump strength.

PubMed Disclaimer

Conflict of interest statement

Competing Interests: The authors have declared that no competing interests exist.

Figures

Figure 1
Figure 1. Schematic representation of the stochastic model.
(a) Scheme of the four-state model for the IP3R and the allowed transitions. The states are represented by Xi, j, k, where the subindex i stands for the IP3 binding site, j for the activator Ca2+ binding site, and k for the inhibitory Ca2+ binding site. For each binding site, a subindex value equal to 1 represents an occupied site, otherwise the site is unoccupied. ki, i = ±1, ±2, ±3, is the rate constant of each state transition. (b) Representation of the states and allowed transitions of the IP3R, which can be inactive (I) (in states X0,0,0, X1,0,0 and X1,1,1) or active (A) (state X1,1,0). (c) Example of some configurations of the four-subunit channel (we show just 4 of the 256 possible configurations for the channel). The channel is open (indicated by O) only when 3 or 4 subunits are in the active state, otherwise it is closed (C).
Figure 2
Figure 2. Analysis of spatial-temporal profiles.
A: Calcium density plot showing a typical outcome of the model simulation (horizontal axes: space, vertical axes: time). B: Thresholded image, black spots are regions where the Ca+2 level exceeds 0.3 μM. C: All spots shown in B are counted and characterized (intensity, size, duration and velocity, i.e., angle α).
Figure 3
Figure 3. Typical outcomes of the model simulation.
Calcium density in fragments of space (horizontal) × time (vertical) for d = 2.5 μm. Whereas for p = 1.8 μM/s different kinds of release events coexist (A), for p = 1.6 μM/s global release events are prevalent (B). Two fronts annihilate each other upon collision (white star in B).
Figure 4
Figure 4. Statistical analysis of Ca2+ waves.
(A) Number of Ca2+ wave release events vs. intercluster distance for different values of pump strength p. (B) Value of intercluster distance corresponding to the maximum number of Ca2+ waves (d max) as a function of p. (C) Mean wave duration vs. intercluster distance for different values of pump strength p. Waves are classified according to the spatial criteria (more details in the text).
Figure 5
Figure 5. Phase diagram in the d–p space.
The plot shows a region dominated by propagating waves (nw > naw) and a region where the abortive waves are more abundant (naw > nw). Calcium release events are classified according to the spatial criterion (dashed line) and the calcium released criterion (solid line).
Figure 6
Figure 6. CaT for different release events.
Total amount of calcium as a function of the pump strength p and the intercluster distance d. (A) CaT averaged over all events, (B) CaT averaged over puffs, (C) CaT averaged over propagating waves, and (D) CaT averaged over abortive waves. Vertical axes were scaled by a factor 106. Note that the vertical scales are different.
Figure 7
Figure 7. CaT versus the pump strength p.
Total amount of calcium for different values of the intercluster distance d, as indicated. CaT averaged over all events (TOTAL), puffs (PUFFS), propagating waves (W) and abortive waves (AW). Vertical axes were scaled by a factor 106. Note that the vertical scale for the figures is different.
Figure 8
Figure 8. Velocity of calcium waves.
Mean calcium wave velocity (vw) vs. the intercluster distance d and the pump strength p. Blue bullets: estimations obtained from the simulation results. The light-blue plane corresponds to the root square of the fitting: vw2(d,p)=107.43−10.99d−12.19p. The coefficient of determination of the data fitting proposed is R 2 = 0.83.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

References

    1. Ur-Rahman T, Skupin A, Falcke M, Taylor CW (2009) Clustering of InsP3 receptors by InsP3 retunes their regulation by InsP3 and Ca2+. Nature 458: 655–659. 10.1038/nature07763 - DOI - PMC - PubMed
    1. Callamaras N, Marchant JS, Sun XP, Parker I (1998) Activation and co-ordination of InsP3-mediated elementary Ca2+ events during global Ca2+ signals in Xenopus oocytes. Journal of Physiology 509: 81–91. 10.1111/j.1469-7793.1998.081bo.x - DOI - PMC - PubMed
    1. Zhao Q, Yi M, Xia K, Zhan M (2009) Information propagation from IP3 to target protein: A combined model for encoding and decoding of Ca2+ signal. Physica A 388: 4105–4114. 10.1016/j.physa.2009.06.033 - DOI
    1. Jaffe LF (1991) The path of calcium in cytosolic calcium oscillations: a unifying hypothesis. Proceedings of the National Academy of Sciences of the United States of America 88: 9883–9887. 10.1073/pnas.88.21.9883 - DOI - PMC - PubMed
    1. Marchant J, Callamaras N, Parker I (1999) Initiation of IP3-mediated Ca2+ waves in Xenopus oocytes. The EMBO Journal 18: 5285–5299. 10.1093/emboj/18.19.5285 - DOI - PMC - PubMed

Publication types

Grants and funding

This research has been partially supported by CONICET (Grant: PIP 0143). The funders had no role in study design, data collection and analysis, decision to publish, or preparation of the manuscript.

LinkOut - more resources