Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2011 Feb 11:10:35.
doi: 10.1186/1475-2875-10-35.

Development of a new version of the Liverpool Malaria Model. I. Refining the parameter settings and mathematical formulation of basic processes based on a literature review

Volker Ermert  1 , Andreas H Fink, Anne E Jones, Andrew P Morse
Affiliations

Development of a new version of the Liverpool Malaria Model. I. Refining the parameter settings and mathematical formulation of basic processes based on a literature review

Volker Ermert et al. Malar J. .

Abstract

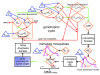
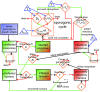
Background: A warm and humid climate triggers several water-associated diseases such as malaria. Climate- or weather-driven malaria models, therefore, allow for a better understanding of malaria transmission dynamics. The Liverpool Malaria Model (LMM) is a mathematical-biological model of malaria parasite dynamics using daily temperature and precipitation data. In this study, the parameter settings of the LMM are refined and a new mathematical formulation of key processes related to the growth and size of the vector population are developed.

Methods: One of the most comprehensive studies to date in terms of gathering entomological and parasitological information from the literature was undertaken for the development of a new version of an existing malaria model. The knowledge was needed to allow the justification of new settings of various model parameters and motivated changes of the mathematical formulation of the LMM.

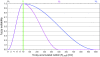
Results: The first part of the present study developed an improved set of parameter settings and mathematical formulation of the LMM. Important modules of the original LMM version were enhanced in order to achieve a higher biological and physical accuracy. The oviposition as well as the survival of immature mosquitoes were adjusted to field conditions via the application of a fuzzy distribution model. Key model parameters, including the mature age of mosquitoes, the survival probability of adult mosquitoes, the human blood index, the mosquito-to-human (human-to-mosquito) transmission efficiency, the human infectious age, the recovery rate, as well as the gametocyte prevalence, were reassessed by means of entomological and parasitological observations. This paper also revealed that various malaria variables lack information from field studies to be set properly in a malaria modelling approach.

Conclusions: Due to the multitude of model parameters and the uncertainty involved in the setting of parameters, an extensive literature survey was carried out, in order to produce a refined set of settings of various model parameters. This approach limits the degrees of freedom of the parameter space of the model, simplifying the final calibration of undetermined parameters (see the second part of this study). In addition, new mathematical formulations of important processes have improved the model in terms of the growth of the vector population.

PubMed Disclaimer

Figures

Figure 1
Figure 1
Components of the LMM. Illustration of various components of the LMM version of 2010. Blue and red arrows depict the rainfall and temperature dependence of various parts of the model, respectively. The fuzzy logic approach of the oviposition as well as the immature mosquito survival are displayed by pink arrows. Note that abbreviations of model parameters are explained in Table 1.
Figure 2
Figure 2
Fuzzy distribution model. Illustration of the fuzzy function with regard to the influence of the 10-day accumulated rainfall (RΣ10d) on the number of oviposited eggs per female mosquito (#Eo) as well as the daily immature mosquito survival probability (ηd). The green vertical line at 10 mm (= S) depicts the most suitable rainfall conditions and separates different scales of the abscissa. Pink and blue lines depict two different settings of the fuzzy distribution model. According to these adjustments rainfall condition are unsuitable for RΣ10d values of 0 mm (= U1) and above of 500 or 1000 mm (= U2), respectively.
Figure 3
Figure 3
Mosquito survival schemes. Illustration of different schemes regarding the daily mosquito survival (pd) against the daily mean temperature (T): the Lindsay-Birley (humid (dry) conditions in dashed purple (orange)), the Martens I (red line; derived from [57-59]), the Martens II (green line; given by [27] and [59]), and the Bayoh scheme (blue line; derived from [61]). Crosses (+) denote pd values with regard to different temperature and humidity conditions (see text). In addition, the data basis of the two Martens schemes is inserted as dots (•).
Figure 4
Figure 4
Flow chart of the simulation of the mosquito population. Flow chart of various components of the LMM version of 2010 regarding the simulation of the mosquito population. The gonotrophic cycle as well as the development of immature mosquitoes within the aquatic stages are illustrated. Individual states of immature and mature mosquitoes are indicated by black rectangles. The orange rhombi denote decisions within the model as well as implemented functions. Green and red arrows represent a positive and negative affirmation, respectively. The impact of the model drivers is indicated via blue triangles and blue arrows (T: daily mean temperature; RΣ10d: 10-day accumulated rainfall). Note that abbreviations of model parameters are explained in Table 1.
Figure 5
Figure 5
Flow chart of the malaria parasite transmission between humans and mosquitoes. Flow chart of various components of the LMM version of 2010 in terms of the modelling of the malaria parasite transmission between the human and mosquito populations. The sporogonic cycle of infected female mosquitoes is furthermore displayed. Individual states of humans and mosquitoes are indicated by black rectangles. The orange rhombi denote decisions within the model as well as implemented functions. Green and red arrows represent a positive and negative affirmation, respectively. The impact of model drivers is indicated via blue triangles and blue arrows (T: daily mean temperature; RΣ10d: 10-day accumulated rainfall; trim: trickle of the number of added infectious mosquitoes). Note that abbreviations of model parameters are explained in Table 1.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Githeko AK, Lindsay SW, Confalonieri UE, Patz JA. Climate change and vector-borne diseases: a regional analysis. Bull World Health Org. 2000;78:1136–1147. - PMC - PubMed
    1. Martens WJM, Jetten TH, Focks DA. Sensitivity of malaria, schistomiasis and dengue to global warming. Clim Change. 1997;35:145–156. doi: 10.1023/A:1005365413932. - DOI
    1. Patz JA, Strzepec K, Lele S, Hedden M, Green S, Noden B, Hay SI, Kalkstein L, Beier JC. Predicting key malaria transmission factors, biting and entomological inoculation rates, using modelled soil moisture in Kenja. Trop Med Int Health. 1998;3:818–827. doi: 10.1046/j.1365-3156.1998.00309.x. - DOI - PubMed
    1. Ross R. The prevention of malaria. 2. London, United Kingdom: John Murray; 1911.
    1. Dietz K, Molineaux L, Thomas A. A malaria model tested in the African savannah. Bull World Health Org. 1974;50:347–357. - PMC - PubMed

Publication types