Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2010 Dec 15;99(12):3916-22.
doi: 10.1016/j.bpj.2010.10.043.

Single-molecule adhesion forces and attachment lifetimes of myosin-I phosphoinositide interactions

Serapion Pyrpassopoulos  1 , Henry Shuman, E Michael Ostap
Affiliations

Single-molecule adhesion forces and attachment lifetimes of myosin-I phosphoinositide interactions

Serapion Pyrpassopoulos et al. Biophys J. .

Abstract

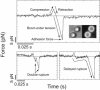
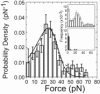
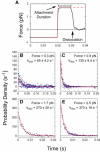
Phosphoinositides regulate the activities and localization of many cytoskeletal proteins involved in crucial biological processes, including membrane-cytoskeleton adhesion. Yet little is known about the mechanics of protein-phosphoinositide interactions, or about the membrane-attachment mechanics of any peripheral membrane proteins. Myosin-Ic (myo1c) is a molecular motor that links membranes to the cytoskeleton via phosphoinositide binding, so it is particularly important to understand the mechanics of its membrane attachment. We used optical tweezers to measure the strength and attachment lifetime of single myo1c molecules as they bind beads coated with a bilayer of 2% phosphatidylinositol 4,5-bisphosphate and 98% phosphatidylcholine. Adhesion forces measured under ramp-load ranged between 5.5 and 16 pN at loading rates between 250 and 1800 pN/s. Dissociation rates increased linearly with constant force (0.3-2.5 pN), with rates exceeding 360 s(-1) at 2.5 pN. Attachment lifetimes calculated from adhesion force measurements were loading-rate-dependent, suggesting nonadiabatic behavior during pulling. The adhesion forces of myo1c with phosphoinositides are greater than the motors stall forces and are within twofold of the force required to extract a lipid molecule from the membrane. However, attachment durations are short-lived, suggesting that phosphoinositides alone do not provide the mechanical stability required to anchor myo1c to membranes during multiple ATPase cycles.

PubMed Disclaimer

Figures

Figure 1
Figure 1
Representative examples of ramp-load rupture events. (Top) The three characteristic regions are: compression of the bead on the pedestal, retraction of the bead in the opposite direction until the compressive force reaches zero, and bond under linearly increasing tension until rupture. (Inset) Transmitted and fluorescence micrographs showing 1-μm-diameter beads coated with 2% PtdIns(4,5)P2, 97.5% DOPC, and 0.5% LRPE (1,2-dioleoyl-sn-glycero-3-phosphoethanolamine-n-(lissamine rhodamine B sulfonyl)). (Bottom, left) Double rupture and (bottom, right) delayed rupture events made up <10% of interactions and were excluded from the analysis.
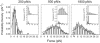
Figure 2
Figure 2
Probability density distributions of forces required to dissociate myo1cIQ-tail from supported lipid membranes containing 2% PtdIns(4,5)P2 at loading rates of 250 ± 29, 930 ± 120, and 1800 ± 230 pN/s (standard deviation). The corrected distributions in the main panels were obtained by subtracting (inset, bottom) frequency distributions (per contact cycle) obtained in the absence of myo1cIQ-tail from (inset, top) uncorrected frequency distributions. (Solid lines) Best fits of the distributions to a model for a single transition barrier (Eq. 1). The fitting results are presented in Table 1, and information regarding numbers of cycles and interactions are presented in Table S1. Errors are standard deviations calculated from bootstrap data sets.
Figure 3
Figure 3
Probability density distribution of forces required to extract a biotinylated-lipid from supported lipid membranes using neutravidin-coated pedestals at a loading rate of 1100 ± 130 pN/s (standard deviation). The corrected distribution was obtained by subtracting (inset, bottom) the frequency distribution acquired in control experiments that did not contain neutravidin from (inset, top) the uncorrected frequency distribution. (Solid lines) Best fit of the distribution to a model for a single transition barrier (Eq. 1). (Dashed line) Best fit of a model that assumes the rupture peaks correspond to single or simultaneous double-bond ruptures (Eq. 5). The fitting results are presented in Table 1. Errors are standard deviations calculated from bootstrap data sets.
Figure 4
Figure 4
Measurement of myo1cIQ-tail-membrane dissociation rates under constant tension. (A) The attachment duration of a single attachment under 1.7 pN of load. A square pulse (dashed trace) is the command to drive compression and retraction of the membrane-coated bead. Positive forces on the bond (solid trace) are recorded during attachments. When a bond is formed between the membrane-coated bead and the myo1cIQ-tail, a constant separating force is maintained via a feedback loop until the bond ruptures. (B–E) Normalized survival probability density of the bond as a function of attachment duration under constant tension is plotted. (Solid lines) Fitting curves of the survival probability densities to Eq. 7. Information regarding number of cycles and interactions are presented in Table S2.
Figure 5
Figure 5
Plot of the dissociation rate of myo1cIQ-tail from 2% PtdIns(4,5)P2-containing membranes as a function of applied separating force (open, red circles). The dissociation rate of myo1cIQ-tail measured at zero force via stopped-flow experiments (17) is shown as a star. A weighted linear fit (solid, red line) gives a slope of 150 ± 10 s−1 pN−1 and a y intercept of 0.45 ± 2.7 s−1 (correlation coefficient = 0.998). Force-dependences of dissociation rates were calculated (Eq. 6) from ramp force histograms obtained at loading rates of (open, black squares) 250, (open, green circles) 930, and (open, blue triangles) 1800 pN/s. The force dependence of the rate of lipid extraction from the membrane at loading rate of 1100 pN/s was also calculated (open, magenta diamonds) from the ramp force distribution. Force-dependent dissociation rates derived from parameters obtained from the best-fits of the corresponding ramp-load experiments (Table 1) are plotted (dashed curves of the corresponding color) using Eq. 2.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Yin H.L., Janmey P.A. Phosphoinositide regulation of the actin cytoskeleton. Annu. Rev. Physiol. 2003;65:761–789. - PubMed
    1. Sheetz M.P., Sable J.E., Döbereiner H.G. Continuous membrane-cytoskeleton adhesion requires continuous accommodation to lipid and cytoskeleton dynamics. Annu. Rev. Biophys. Biomol. Struct. 2006;35:417–434. - PubMed
    1. Mashanov G.I., Tacon D., Molloy J.E. The spatial and temporal dynamics of pleckstrin homology domain binding at the plasma membrane measured by imaging single molecules in live mouse myoblasts. J. Biol. Chem. 2004;279:15274–15280. - PubMed
    1. Hokanson D.E., Laakso J.M., Ostap E.M. Myo1c binds phosphoinositides through a putative pleckstrin homology domain. Mol. Biol. Cell. 2006;17:4856–4865. - PMC - PubMed
    1. Spudich G., Chibalina M.V., Kendrick-Jones J. Myosin VI targeting to clathrin-coated structures and dimerization is mediated by binding to Disabled-2 and PtdIns(4,5)P2. Nat. Cell Biol. 2007;9:176–183. - PMC - PubMed

Publication types

LinkOut - more resources