Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2001 Sep 25;98(20):11324-9.
doi: 10.1073/pnas.181338798.

Self-organization of a propulsive actin network as an evolutionary process

I V Maly  1 , G G Borisy
Affiliations

Self-organization of a propulsive actin network as an evolutionary process

I V Maly et al. Proc Natl Acad Sci U S A. .

Abstract

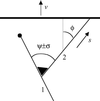
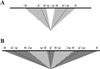
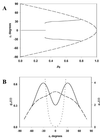
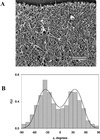
The leading edge of motile cells is propelled by polymerization of actin filaments according to a dendritic nucleation/array treadmilling mechanism. However, little attention has been given to the origin and maintenance of the dendritic array. Here we develop and test a population-kinetics model that explains the organization of actin filaments in terms of the reproduction of dendritic units. The life cycle of an actin filament consists of dendritic nucleation on another filament (birth), elongation by addition of actin subunits and, finally, termination of filament growth by capping protein (death). The regularity of branch angle between daughter and mother filaments endows filaments with heredity of their orientation. Fluctuations of branch angle that become fixed in the actin network create errors of orientation (mutations) that may be inherited. In our model, birth and death rates depend on filament orientation, which then becomes a selectable trait. Differential reproduction and elimination of filaments, or natural selection, leads to the evolution of a filament pattern with a characteristic distribution of filament orientations. We develop a procedure based on the Radon transform for quantitatively analyzing actin networks in situ and show that the experimental results are in agreement with the distribution of filament orientations predicted by our model. We conclude that the propulsive actin network can be understood as a self-organizing supramolecular ensemble shaped by the evolution of dendritic lineages through natural selection of their orientation.

PubMed Disclaimer

Figures

Figure 1
Figure 1
Organizational unit of actin network at the leading edge. Near the leading edge (heavy line), filaments (solid lines) are nucleated on existing ones by the Arp2/3 complex (triangle). The angle between the mother (1) and daughter (2) filaments is ψ on average, with root-mean-square deviation σ. Filaments elongate and propel the plasma membrane and are stochastically terminated by capping protein (solid circle). Orientation of a filament is characterized by its incidence angle with the leading edge, φ (φ as shown is positive). Velocity of membrane advance is denoted by v; rate of filament elongation is denoted by s, which depends on φ.
Figure 2
Figure 2
Diagrams showing reproductive patterns of actin filaments at the leading edge. Heavy lines represent the leading edge. Angles noted are the incidence angles of lines and boundaries with respect to the leading edge. Hatched and crosshatched areas represent the range of the incidence angle φ, within which a filament is reproductively successful. The branching angle, ψ, is shown equal to 67°. (A) When the critical angle θ is larger than ψ/2 but smaller than ψ, two orientations alternate in successive generations. Dashed lines show an example of a pair of such orientations (φ′, φ′ + ψ). Filaments with these orientations form a particular two-orientation type. (B) When the critical angle θ is larger than ψ, in successive generations there can be three orientations (in the crosshatched area) as well as two (in the hatched area). Dashed lines show an example of a triplet of orientations (φ′ − ψ, φ′, φ′ + ψ). Filaments that have these orientations form a particular three-orientation type.
Figure 3
Figure 3
Steady-state density (relative frequency) qss(φ) of filaments with the incidence angle φ at the leading edge. (A) Locations of the maxima of qss(φ) (solid lines) are incidence angles of the most abundant filaments under different p0, the probability that the filament with φ = 0 is not obstructed by the plasma membrane. Beyond the dashed line, qss(φ) is not defined, that is, existence of such angles is not predicted. (B) qss(φ) for p0 = 0.2 (dashed curve, left axis), p0 = 0.5 (solid curve, left axis), and p0 = 0.9 (dotted curve, right axis). ψ = 67°, σ = 12°.
Figure 4
Figure 4
Orientation of actin filaments at the leading edge. (A) Platinum replica electron micrograph of the dendritic brush of actin filaments at the leading edge of a moving Xenopus keratocyte. The cell margin is at the top of the image. (Bar = 0.2 μm.) (B) Histogram of angles between filaments and the normal to the leading edge in the actin cytoskeleton of a Xenopus keratocyte lamellipodium (bars) and its theoretical approximation (curve). Negative and positive angles mean deviation to one and the other side from the normal. The region shown in A corresponds to approximately one-half the lamellipodial area from which the histogram was generated. The histogram is representative of histograms obtained from other regions of lamellipodia and other cells.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Pollard T D, Blanchoin L, Mullins R D. Annu Rev Biophys Biomol Struct. 2000;29:545–576. - PubMed
    1. Small J V, Herzog M, Anderson K. J Cell Biol. 1995;129:1275–1286. - PMC - PubMed
    1. Michod R E. Darwinian Dynamics: Evolutionary Transitions in Fitness and Individuality. Princeton, NJ: Princeton Univ. Press; 1999.
    1. Grant V. The Evolutionary Process: A Critical Review of Evolutionary Theory. New York: Columbia Univ. Press; 1985.
    1. Rowe G W. Theoretical Models in Biology: The Origin of Life, the Immune System, and the Brain. New York: Oxford Univ. Press; 1994.

Publication types

LinkOut - more resources