Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2001 Oct;10(10):2075-82.
doi: 10.1110/ps.09201.

Hydrogen-bonding classes in proteins and their contribution to the unfolding reaction

R Ragone  1
Affiliations

Hydrogen-bonding classes in proteins and their contribution to the unfolding reaction

R Ragone. Protein Sci. 2001 Oct.

Abstract

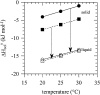
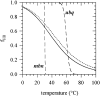
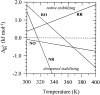
This paper proposes to assess hydrogen-bonding contributions to the protein stability, using a set of model proteins for which both X-ray structures and calorimetric unfolding data are known. Pertinent thermodynamic quantities are first estimated according to a recent model of protein energetics based on the dissolution of alkyl amides. Then it is shown that the overall free energy of hydrogen-bond formation accounts for a hydrogen-bonding propensity close to helix-forming tendencies previously found for individual amino acids. This allows us to simulate the melting curve of an alanine-rich helical 50-mer with good precision. Thereafter, hydrogen-bonding enthalpies and entropies are expressed as linear combinations of backbone-backbone, backbone-side-chain, side-chain-backbone, and side-chain-side-chain donor-acceptor contributions. On this basis, each of the four components shows a different free energy versus temperature trend. It appears that structural preference for side-chain-side-chain hydrogen bonding plays a major role in stabilizing proteins at elevated temperatures.

PubMed Disclaimer

Figures

Fig. 1.
Fig. 1.
Solution enthalpy of isomeric solid and liquid N-alkyl amides. The packing contribution to the dissolution process can be easily estimated, assuming that the enthalpy of the solid isomers can be dissected according to the scheme solid → liquid → water, where the liquid-to-water step is given by the liquid isomers. The fusion enthalpy, indicated by the arrows, is given by the difference between solution enthalpies of N-t-butyl-acetamide (solid circles) or 2,2,N-trimethyl-propanamide (solid squares) and their liquid isomers, N-butyl-acetamide (open circles) or N-methyl-pentanamide (open squares), 8.81 and 12.12 kJ/mole at 25°C, respectively, and is obtained using the original thermochemical data (Konicek and Wadsö 1971; Sköld et al. 1976 ).
Fig. 2.
Fig. 2.
Unfolding curve of a helical 50-mer. The experimental helical fraction (fHB) of the alanine-rich peptide (solid line) was obtained using a van't Hoff enthalpy (δHvH) of 46 kJ/mole (corresponding to 11 kcal/mole) and a midpoint temperature of 314 K (Scholtz et al. 1991). The theoretical unfolding curve (dashed line) was drawn with the assumption that δGHB° = 5.26 − T × 0.0165 (in units of kJ/mole) represents the free energy for disrupting a single hydrogen bond. δGHB° was then multiplied by 50, to account for length, and divided by 6, to account for deviation from a two-state reaction, according to the calorimetric to van't Hoff enthalpy ratio originally found. The midpoint temperature is ∼319 K. Long dashed curves refer to myoglobin and ubiquitin (mbn and ubq, respectively) and were obtained using values of δH376 and δS385 reported in Table 1.
Fig. 3.
Fig. 3.
Contribution of hydrogen bonds to protein unfolding. Hydrogen bonds were classified as backbone donor–to–backbone acceptor (NO), side-chain donor–to–backbone acceptor (RO), backbone donor–to–side-chain acceptor (NR), side-chain donor–to–side-chain acceptor (RR) bonds. Lines were drawn according to equation 5 (see Materials and Methods), using fitting parameters thus obtained, i.e., δhNO = (1.9 ± 0.7), δhRO = (12.3 ± 4.6), δhNR = (29.5 ± 5.7), and δhRR = (−4.2 ± 4.5) kJ/mole for the enthalpy (r = 0.978), and δsNO = (6.5 ± 2.2), δsRO = (34.8 ± 15.6), δsNR = (93.4 ± 19.2), and δsRR = (−15.2 ± 15.3) J/mole per K for the entropy (r = 0.975).
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Baldwin, R.L. 1986. Temperature dependence of the hydrophobic interaction in protein folding. Proc. Natl. Acad. Sci. 83 8069–8072. - PMC - PubMed
    1. Baldwin, R.L. and Muller, N. 1992. Relation between the convergence temperatures Th* and Ts* in protein unfolding. Proc. Natl. Acad. Sci. 89 7110–7113. - PMC - PubMed
    1. Becktel, W.J. and Schellman, J.A. 1987. Protein stability curves. Biopolymers 26 1859–1877. - PubMed
    1. Chen, C.-C., Zhu, Y., King, J.A., and Evans, L.B. 1992. A molecular thermodynamic approach to predict the secondary structure of homopolypeptides in aqueous systems. Biopolymers 32 1375–1392. - PubMed
    1. Costas, M., Kronberg, B., and Silveston, R. 1994. General thermodynamic analysis of the dissolution of non-polar molecules into water. J. Chem. Soc., Faraday Trans. 90 1513–1522.

Publication types

LinkOut - more resources