Abstract
Molecular dynamics simulations reveal substructures within the liquid-ordered phase of lipid bilayers. These substructures, identified in a 10 μsec all-atom trajectory of liquid-ordered/liquid-disordered coexistence (Lo/Ld), are composed of saturated hydrocarbon chains packed with local hexagonal order, and separated by interstitial regions enriched in cholesterol and unsaturated chains. Lipid hydrocarbon chain order parameters calculated from the Lo phase are in excellent agreement with 2H NMR measurements; the local hexagonal packing is also consistent with 1H-MAS NMR spectra of the Lo phase, NMR diffusion experiments, and small angle X-ray- and neutron scattering. The balance of cholesterol-rich to local hexagonal order is proposed to control the partitioning of membrane components into the Lo regions. The latter have been frequently associated with formation of so-called rafts, platforms in the plasma membranes of cells that facilitate interaction between components of signaling pathways.
Keywords: lipid rafts, cholesterol, molecular dynamics, Hidden Markov Model
INTRODUCTION
Two phase coexistence in binary mixtures of a lipid with cholesterol was first observed in the early 1970s.1 More recently, coexisting liquid phases, known as “liquid ordered” (Lo) and “liquid disordered” (here called Ld, sometimes called Lα) in cholesterol containing mixtures of three components have been studied extensively.2–4 The Lo phase5 shares features with raft domains in cell membranes6–8 — it is enriched in saturated lipids and is cholesterol dependent. Experimental evidence for rafts in resting cell membranes is consistent with sizes less than 30 nm.9,10 Nanometer scale Lo domains are found near a miscibility critical point;11–13 nanoscale domains are also observed in lipid mixtures containing lipids with a single unsaturated chain.14–17 However, it is almost universally reported that membrane components which partition into the raft phase in the cell membrane partition out of the Lo phase in ternary mixtures.6
Despite a wealth of experimental data, the detailed molecular structure of nanoscale domains in the Lo/Ld two-phase region remains unknown. Ultimately, molecular scale structure and interactions drive partitioning of membrane components, and knowledge of them will help establish connections between the Lo phase in model membranes and rafts in live cells. The goal of this work is therefore to elucidate the molecular structure of the Lo phase.
An extensive body of molecular dynamics (MD) simulation literature has considered the effect of cholesterol on bilayers (see review by Róg, et al.18), including recent simulations of binary19–22 and ternary mixtures.23–25 While an important first step, these results cannot provide strong evidence for liquid-liquid phase separation, since conventional “all-atom” MD timescales have so far been insufficient to sample lipid mixing degrees of freedom. This is clear, based on the diffusion of individual lipids — on the timescale of 100 nsec, a lipid with a diffusion coefficient of 10−7 cm2/sec covers an area of 12 nm2 which is significantly smaller than the size of a typical membrane patch in simulations. Furthermore, lateral heterogeneity of lipid distribution may slow mixing relative to this naive estimate.26 However, on the timescale of 10 μsec, as used in this study, an area of 1.2 μm2 is covered which is much larger and potentially sufficient to achieve an equilibrium lateral distribution of lipids. (Limited mixing of lipid components, may be effected by advanced techniques,27 at the expense of realistic dynamics.)
Ten μsec all atom simulations of a ternary mixture of dioleyoylphosphatidylcholine (DOPC), dipalmitoylphosphatidylcholine, (DPPC), and cholesterol obtained on the An-ton special purpose computer28 are presented. At a temperature just below the miscibility transition (denoted by Tm), robust Lo/Ld coexistence is observed despite extensive lipid mixing, and is confirmed by comparison of lipid chain order parameters from the simulation to measured 2H NMR quadrupolar splittings and by control simulations of the homogeneous phases. The simulations reveal previously unobserved substructure within the Lo phase comprised of saturated chains packed in transient regions of local hexagonal order. The substructure within the Lo phase suggests an alternate view on the molecular mechanisms that drive liquid-liquid phase separation, and provides insight into partitioning of membrane components into the Lo phase.
METHODS AND MODELS
Building the initial configuration
Two separate bilayer systems were built with the CHARMM-GUI, one with an Lo composition and one with an Ld composition.29 The compositions were taken from published experimental results for a 1:1 ratio of DOPC:DPPC + 20 mol % chol at 298 K.13 The Lo system comprised 76 DOPC, 280 DPPC, and 156 CHOL, and the Ld system 240 DOPC, 116 DPPC, and 44 CHOL. Each bilayer was simulated in the constant particle number, pressure, and temperature ensemble (NPT) until the membrane area equilibrated. The initial configuration for the Lo/Ld system was then created by embedding a 5 nm diameter, roughly circular patch of the equilibrated Lo bilayer into the equilibrated Ld bilayer, and eliminating any overlapping lipids from the Ld bilayer (Figure S1). Care was taken both to maintain the correct composition of each region and to obtain a symmetric bilayer during this process. After embedding the Lo domain and symmetrizing the bilayer, the final number of lipids of each type was 174 DOPC, 114 DPPC, and 60 CHOL. This gives a bulk composition that is closer to 1:1.5 +20% DOPC:DPPC + CHOL, but since the miscibility transition temperature and compositions of each phase are only weakly dependent on the DOPC fraction,13 we expect this deviation to have a minor effect. A water box containing 16,261 waters and 0.150 M NaCl was added. The resulting system was allowed to relax under NPT conditions again, while weakly restraining the lipid headgroups to maintain the monolayers and also prevent water from entering the gap between the Lo and Ld regions. After the system area had relaxed, the restraints were removed, and system was relaxed for just over 25 nanoseconds of unrestrained NPT simulation. The end result of this procedure is shown in Figure S1, panel C. An initial configuration for a control simulation for the high temperature, mixed phase was built with the CHARMM-GUI, this time simply choosing the positions of all three components randomly.
Production simulation on Anton
Anton is a special purpose machine designed for high performance molecular dynamics calculations. Details on the Anton machine can be found elsewhere 30. The equations of motion were integrated with the Verlet algorithm with a timestep of 2.0 fsec. A constant temperature and a pressure of 1 atm were maintained by the Martyna-Tobias-Klein method,31 with the pressure coupling effected every 240 fsec and the temperature coupling every 24 fsec. The temperature of the system initiated from a phase separated state was maintained at 298 K, the temperature of the homogeneous system was maintained at 328 K. Lennard-Jones interactions were truncated at 10.14 Å by a hard cutoff with no shift. Long range electrostatics were computed by the k-space Gaussian split Ewald method32 on a 64×64×64 point grid, with the parameters of the Gaussian chosen to yield a root mean squared error in the electrostatic force calculation of 0.18%. The duration of the T = 298 K system was 9.4 μsec, the duration of the T = 328 K system was 6.9 μsec, and the duration of the Lo control was 5.0 μsec.
Local composition analysis
We calculated the locally averaged area density of DPPC on a 20 × 20 grid (roughly 5 Å between grid points), counting DPPC phosphates within 10 Å of each grid point for each simulation snapshot. This quantity we call ρ(r), where r labels the grid point. To obtain a range for this observable that crosses zero at the average and has a range of order 1, we rescale this quantity by subtracting and dividing by the bulk average of ρ(r): ρ̃(r) ≡ (ρ(r)− 〈ρ〉)/〈ρ〉, where 〈ρ〉 is area density of DPPC averaged over the entire simulation cell. For this composition, 〈ρ〉 ≅ 0.57nm−2. ρ̃(r) ranges from −1 (no DPPC) to a (positive) maximum value that corresponds to the highest observed local density of DPPC, roughly 4 for both temperatures.
Hidden Markov Model technical details
The HMM consists of two hidden states (putatively Lo and Ld) and 28 emission signals (the 28 different local lipid compositions). For each lipid in each frame of simulation (sampled every 0.239 ns), the local composition is measured. The time-ordered local composition is the emission signal for that unknown state. Between each frame, the lipid may change state (e.g., Lo to Ld) with a probability that does not depend on its history. There are four such so-called transition probabilities; each of the two states has a probability of changing or not changing state.
There are a total of 60 HMM parameters: 28 emission probabilities for each state and two transition probabilities for each state. The probabilities are constrained to sum to one, yielding 56 unique values to determine. These are determined by the Baum-Welch algorithm.33,34 For some initial “guess” parameters, the algorithm computes the probability of observing the composition sequence given all possible sequences of states (summation over the astronomical number of paths is simplified by taking advantage of the Markovian character of the system via a forward-backward algorithm). The total expected number of emissions and transitions that occurred (summing over each possible path) are normalized and used as probabilities for the next iteration. Although an iteration is guaranteed to produce a more likely HMM, convergence to a global maximum is not guaranteed. Convergence of the parameters was checked by starting from ten initial guesses and selecting the best solution. In most cases a majority of the runs converged to nearly the same (most probable) model.
Once the HMM parameters are determined, the most likely state sequence is determined by the Viterbi algorithm,35 which iterates through a sequence, saving (at each frame) the most likely state path to that frame from the start. Again, Markovian character is crucial to calculate the probability of a longer state sequence from the previous one.
A comparison of the HMM state assignment to the local density of DPPC and the thickness in each leaflet is shown for several configurations for T < Tm (Fig. S2) and for T > Tm (Fig. S3).
Calculation of deuterium order parameters and quadrupolar spectra
Deuterium order parameters were calculated at each position along the aliphatic chains of DPPC according to
where θ is the angle between the carbon – hydrogen bond vector and the membrane normal, and the average is taken over snapshots separated by 2.4 nsec. The lipids are divided into two populations for the calculation based on the Hidden Markov Model analysis. The left and right panels of Figure S4 present the order parameters for the T < Tm and T > Tm system, respectively.
Calculation of 2H NMR spectra
The simulated spectra were calculated according to the formula
where Δνq(n) are the quadrupolar splittings for each methylene- and methyl group of sn-1 and sn-2 hydrocarbon chains, e2qQ/h=167 kHz, and S(n) are the order parameters from the simulation. A random distribution of orientations of bilayer normals to the magnetic field was assumed.
Extraction of SCD values from experimentally measured 2H spectra
The order parameters of hydrocarbon chains of DPPC in the spectrum of coexisting Ld and Lo phases were extracted by choosing a pure Ld phase spectrum recorded at higher temperature that perfectly matched the Ld component in the Ld/Lo spectrum. After intensity adjustment, this Ld spectrum was subtracted to yield the spectrum of the pure Lo component. Order parameters were extracted from the Ld and Lo spectra by integration as described earlier.36 A few resolved quadrupolar splittings were assigned directly. The quality of order parameter extraction was verified by comparing measured and simulated spectra of Ld/Lo phase coexistence.
Calculation of lipid-lipid pair contact frequencies
Lipid-lipid and lipid-cholesterol boundaries are mapped by identifying pairs that share a boundary in a Voronoi tessellation of a configuration. The sample of configurations then provides information on the statistics of pairwise contacts. The extent to which the observed interaction statistics deviate from a random mixture is found by comparison to randomized configurations, under the constraint that the composition remains fixed. Two null hypotheses were considered: Randomization over the entire system, and randomization within each phase.
RESULTS
Lipids mix laterally on 10 μsec timescales
An initial configuration for a Lo nanodomain was prepared by embedding a 5 nm diameter Lo region in a 10 nm × 10 nm Ld bilayer (Fig. S1). The compositions of the two phases were set to the experimentally determined compositions for a ternary mixture of DOPC/DPPC/cholesterol just below the miscibility transition.13 (Details on the system set up and simulation protocol are found in Supplementary Materials (SM).) Fig. 1 illustrates the extent to which the lipids initially in the Lo domain have mixed by the end of the simulation. This may be quantified by asking the question: what is the probability of finding an original Lo lipid at a distance r from another original Lo lipid? Comparing cumulative distribution functions for these lipids (CDF) averaged over the first 100 nsec of the simulation and the last 100 nsec, it is clear that the initial domain of Lo lipids has dispersed (Fig. S2). Furthermore, comparison to the CDF of an equal number of randomly selected lipids shows that by the end of the simulation the initially Lo lipids have mixed (dotted line in Fig. S2), indicating that the simulation time-scale is sufficient to sample compositional degrees of freedom.
Figure 1.
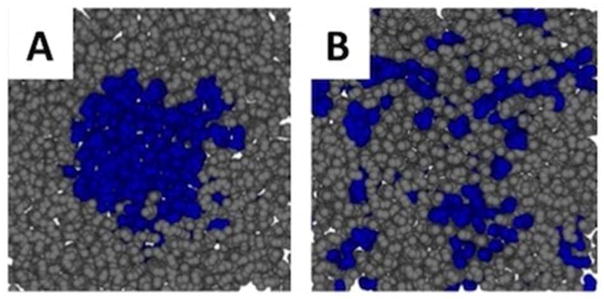
The lipids initially in the Lo domain (blue, Panel A) have mixed by the end of the simulation (Panel B). The cumulative distribution function (Fig. S2) of the lipids initially in the Lo region confirms that by the end of the 10 μsec simulation these lipids are indistinguishable from the same number of randomly chosen lipids.
Local composition reveals a stable domain for T < Tm
Experimentally, it is known that the Lo and Ld regions differ in composition, with the Lo region comparatively enriched in DPPC and cholesterol. Whether a particular lipid belongs to the Lo or the Ld region should therefore be encoded in the composition of its local neighborhood. A neighborhood enriched in DPPC and cholesterol is more likely to be in the Lo phase than Ld, but how much more likely?
This was answered by developing a hidden Markov model (HMM) for the local membrane phase. The local composition in the vicinity of each lipid (determined by the six nearest lipids in the same leaflet, including itself) is recorded in a time series (one for each lipid). This “observable” data is used to define two “hidden” states (putatively Lo or Ld,). The parameters of the model are the probabilities of each hidden state to have a certain lipid composition, and the probabilities for a hidden state to change to the other in one time step. The values of the unknown probabilities were determined by the requirement that they maximize the likelihood of the observed sequence of 39,272 compositions for each of the 348 lipids using standard methods for solving HMMs. The HMM can also be used to define the hidden state at any point in the bilayer, given a local lipid composition. (See Methods for complete details.)
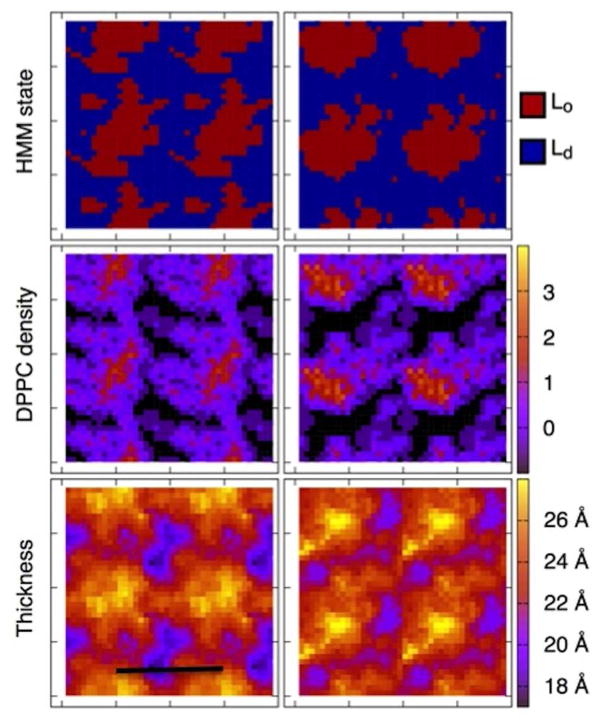
The result of the HMM analysis is a map of each leaflet of the bilayer onto Lo and Ld phases; a representative configuration is shown in the top panels of Fig. 2. The bilayer phase determined by the HMM analysis is consistent with a direct analysis of the local composition and the bilayer thickness (middle and bottom panels of Fig. 2, and S3), both of which are markers of the Lo phase. It is clear that despite extensive mixing of the lipids, a local region with features consistent with the Lo phase persists throughout the simulation.
Figure 2.
Hidden Markov model analysis reveals a robust domain despite extensive lipid mixing. The region identified as Lo by the HMM analysis coincides with a region of enhanced DPPC density (black is no DPPC, and the bulk average DPPC density corresponds to zero on the unitless color scale, defined in Methods), and increased per leaflet membrane thickness. Three periodic images are shown in addition to the simulation cell, the scale bar is 10 nm. Top leaflet is on the left, and bottom is on the right.
The simulation admits direct observation of the dynamics of nanoscale Lo/Ld coexistence, included as a web enhanced object, with the rendering explained in the caption to Figure 4. (“lowT_small.mpeg”) The persistence of the Lo region is apparent, as is the exchange of lipids between the regions. Transient hexagonal structures are observed within the Lo region, discussed in more detail below.
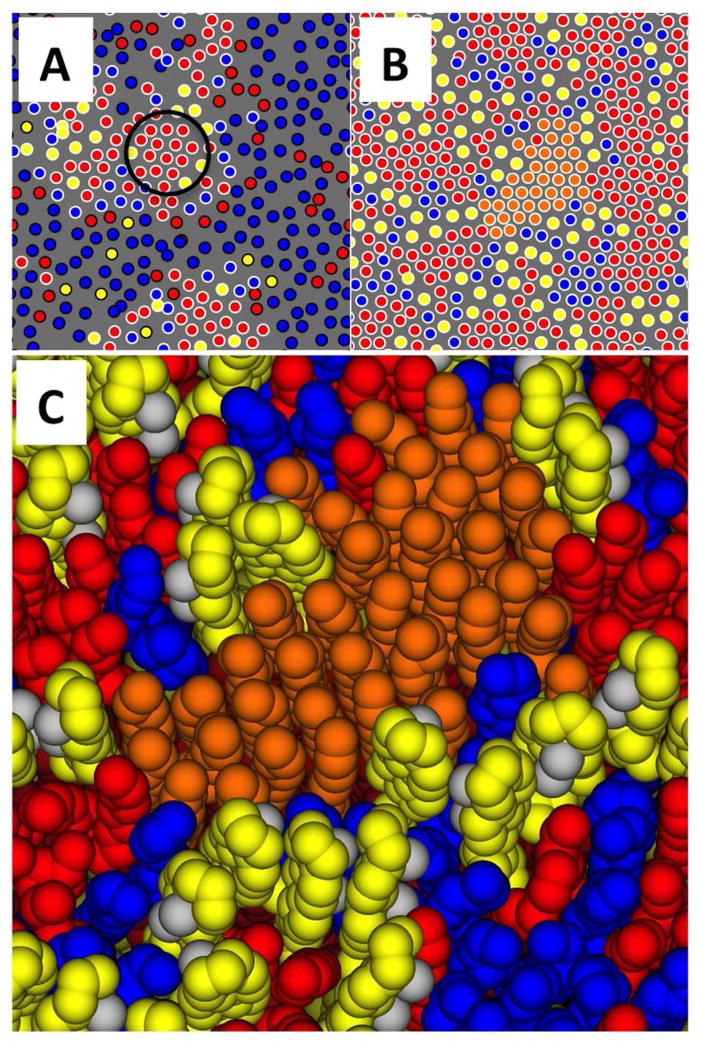
Figure 4.
Center of mass locations of lipid chains and cholesterol for one leaflet of the Lo/Ld simulation at 298 K (A) and the Lo control (B). DPPC chains red, DOPC chains blue, and cholesterol yellow, no periodic images are shown. In panel A, lipids identified as Lo by the HMM analysis are indicated by a white border, and Ld lipids are indicated by a black border. An area of locally hexagonal order is observed within the Lo region, indicated by the black circle. Within such regions, DPPC chains are well-ordered and pack tightly. The control simulation (panel B) reveals multiple locally hexagonal regions, bounded by areas enriched in cholesterol and DOPC. The molecular detail in one such region, highlighted in orange, is shown in panel C. Methyl groups on the β face of the cholesterols are colored gray, headgroups are not rendered in order to reveal the chain structure.
In contrast, a simulation of the homogeneous phase above the miscibility transition temperature reveals compositional fluctuations that at times appear Lo-like, yet occur on a much faster timescale (Fig. S4 and movie available as a web enhanced object “highT_small.mpeg”). Despite a similar structure in the lateral composition, the T > Tm data have distinctly different structure in the hydrocarbon chains, described next.
Deuterium quadrupolar splittings confirm Lo/Ld coexistence
Based on the assignment of each lipid into either the Lo or Ld phase, we computed the C-D order parameters of DPPC hydrocarbon chains (SCD) at each position for both temperatures (Fig. S5). SCD reports the average alignment of the carbon-hydrogen covalent bonds relative to the bilayer normal, and is proportional to the quadropolar splitting of resonances observed in a 2H NMR experiment on samples containing DPPC with perdeuterated hydrocarbon chains. The quadrupolar splittings therefore distinguish the Lo and Ld phases by virtue of the more ordered chains in the Lo phase—indeed, this feature is the origin of the word “ordered” in “liquid ordered phase.”
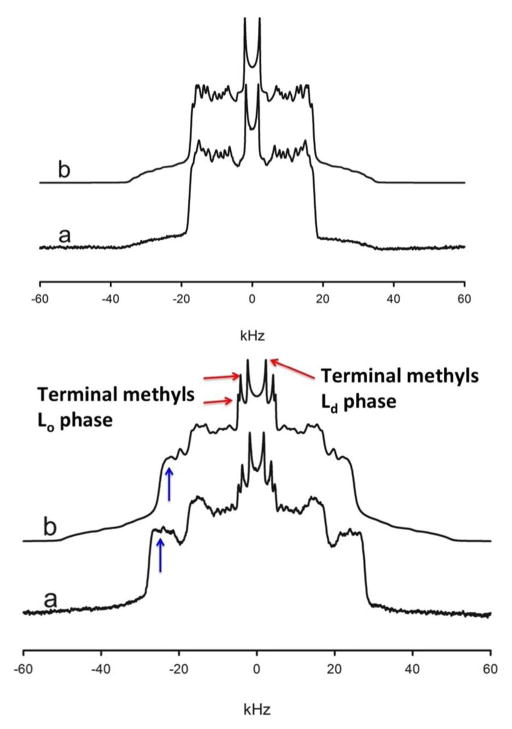
In order to facilitate comparison to the experimental data, 2H NMR spectra were computed from the simulated order parameters as described in Methods. The simulated and measured spectra are shown in Fig. 3. The central resonances corresponding to the terminal methyls (red arrows in the bottom panel of Fig. 3) show a characteristic triple peak structure below Tm, but yield a single splitting above Tm. In the Lo phase (T < Tm), the lipids are oriented parallel to the bilayer normal with more ordered chains. Consequently, the sn-1 chain protrudes more deeply into the bi-layer center than the sn-2 chain, yielding distinct splittings and producing the two outer pairs of peaks below Tm. In the Ld phase the chains are disordered and the terminal methyls produce a single splitting corresponding to the innermost pair of peaks. The resonances arising from the rest of the carbons below Tm overlap and are difficult to distinguish on a carbon-by-carbon basis, but they yield two distinct populations, indicated by the two broad shoulders in the wings of the spectra. By comparison, the high T data in Fig. 3 are consistent with a single population of lipids without any components that are distinguishable on the NMR timescale of about 10 μsec. Table 1 compares the average order parameters from simulation and experiment. The chains of the Lo phase are slightly less ordered on average than reported by the experiment, most likely due to the high fraction of lipids at the Lo/Ld boundary in the T < Tm simulation. Indeed, the average order parameters observed in a control simulation of the Lo phase are in excellent agreement with the experimentally measured values. The compositions of the two phases also agree with those measured experimentally 13 (Table 2), except for the balance of DOPC to DPPC in the Ld phase.
Figure 3.
Comparison of experimental (a) and simulated (b) 2H NMR spectra of DPPC with perdeuterated hydrocarbon chains for T < Tm (bottom panel) and T > Tm (top panel). The low T spectra show the features characteristic of Lo/Ld coexistence. The terminal methyls produce one pair of peaks for the Ld phase (central pair of peaks) and two pairs (one each for the sn-1 and sn-2 chains) for the Lo phase. The rest of the carbons produce two broad bands of peaks arising from overlapping and broadened resonances, with the inner band arising from the Ld phase. The broad shoulder arising from the Lo upper chain methylenes (blue arrows) produce a slightly narrower splitting in the simulated spectrum, indicating slightly less order in the simulation than in the experiment. The best match between the high T simulation data (328 K) and experimental spectrum is found for experimental data acquired at 313 K, indicating a shift in the temperature dependence of the model for T > Tm.
Table 1.
Comparison of experimentally measured and simulated 2H NMR order parameters at 298 K. Order parameters are averaged over all carbons in both sn-1 and sn-2 chains. The experimental order parameters were obtained from the spectra as described in Methods. Standard errors are indicated in the parentheses.
| Expt. | Lo/LdSim. | Control Sim. | |
|---|---|---|---|
| Lo | 0.36 | 0.321(0.003) | 0.373(0.001) |
| Ld | 0.21 | 0.208(0.001) | 0.223(0.001) |
Table 2.
Fractional compositions of the two phases determined by experiment13 and simulation. Standard errors are reported in parentheses.
| CHL | DPPC | DOPC | |
|---|---|---|---|
| Lo (expt.) | 0.31 | 0.55 | 0.15 |
| Lo (sim.) | 0.27(0.2) | 0.51(0.4) | 0.22(0.3) |
| Ld (expt.) | 0.11 | 0.29 | 0.60 |
| Ld (sim.) | 0.08(0.2) | 0.16(0.4) | 0.75(0.5) |
Substructure composed of aligned alkyl chains with locally hexagonal order is observed in the Lo phase
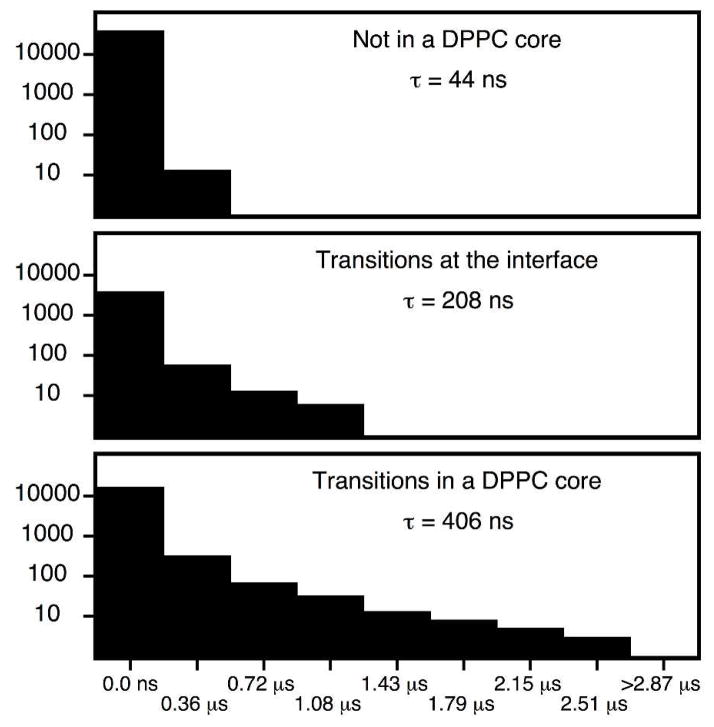
Visualization of the centers of mass of the hydrocarbon chains and cholesterol reveals a local, approximately hexagonal substructure within the Lo phase, comprised of regions of densely packed DPPC chains, shown in Fig. 4A and in the web enhanced object discussed above. Similar substructure is observed in the Lo control simulation (Figs. 4B, 4C). A movie corresponding to Fig. 4B is available as a web enhanced object (“LoControl_small.mpeg”). These regions are composed of lipids with aligned saturated chains oriented parallel to the bilayer normal, as shown in the molecular graphic (Fig. 4C). They have sn-1- and sn-2 hydrocarbon chains with distinctly different quadropolar splittings of terminal methyl groups as measured for the Lo phase. The substructure is nonetheless fluid — the order does not extend over long range, it is dynamic, and analysis of the lifetimes of saturated chain contacts shows that contacts deepest in the hexagonal regions of the Lo domain have a lifetime of approximately 400 nsec (Fig. 5). Despite the compositional fluctuations that mimic the Lo phase, aligned chains, persistent contacts, and locally hexagonal order are not observed above Tm.
Figure 5.
Histograms of aligned chain contact lifetimes. Aligned DPPC chain contacts display a distribution of lifetimes which depends on where the chain contacts are observed. Chain contacts in the locally hexagonal core have the longest lifetime
Because the simulation timescale admits thorough sampling of the compositional degrees of freedom, the statistics of lipid interactions may be addressed directly; here the frequencies of lipid-lipid contacts are analyzed, as measured by boundary lengths in a Voronoi tesselation. These boundary lengths are compared to the expected lengths for random distributions (ideal mixing) over the entire system and within each phase (Table 3, details in Methods). In every case, nearest neighbor cholesterol-cholesterol interactions are strongly disfavored, consistent with previous simulation results for binary mixtures.20 Within the Lo phase, the local hexagonal structure is reflected in the frequency of DPPC-DPPC contacts, which are enhanced relative to ideal mixing. Cholesterol boundaries were distingiushed between the smooth and rough faces (S and R in Table 3). Within the Lo region, cholesterol-DPPC contacts favor the smooth face, while cholesterol-DOPC contacts favor the rough face. Taken together, these data indicate that cholesterol mediates the boundary between the locally hexagonal regions in the Lo phase and the interstitial, more disordered regions, and that it does so by virtue of its two (α and β) faces.
Table 3.
Percentage change in the length of lipid-lipid boundaries, relative to a randomly mixed system. Boundaries with the (S)mooth and (R)ough faces of cholesterol are considered separately. The column labeled Lo/Ld corresponds to the T < Tm phase separated system, Lo is the Lo control, and Ld is the Ld control. The values in parentheses are the standard errors of 10 non overlapping blocks of data.
| Lo/Ld | Lo | Ld | |
|---|---|---|---|
| CHL(S)-DPPC | +31.8(1.2) | +19.0(1.2) | −26.7(1.7) |
| CHL(S)-DOPC | −14.8(1.7) | +6.9(1.7) | +2.0(1.0) |
| CHL(R)-DPPC | +25.2(1.2) | +12.7(1.6) | −28.9(1.2) |
| CHL(R)-DOPC | +0.7(1.3) | +37.1(1.7) | +11.2(1.2) |
| CHL(S)-CHL(S) | +12.6(3.2) | +3.8(1.7) | −54.2(3.2) |
| CHL(S)-CHL(R) | −12.0(2.7) | −18.8(1.7) | −66.0(2.0) |
| CHL(R)-CHL(R) | −42.6(1.7) | −47.1(1.4) | −76.2(1.8) |
| DPPC-DPPC | +25.6(2.8) | +12.8(1.8) | −20.2(1.5) |
| DPPC-DOPC | −25.1(1.7) | −20.3(1.9) | +0.15(0.7) |
| DOPC-DOPC | +19.9(1.2) | −22.3(1.9) | +4.2(0.3) |
DISCUSSION AND CONCLUSIONS
The simulation data reveal substructure within the Lo phase—local regions of hexagonally ordered saturated chains are separated by interstitial regions enriched in cholesterol. This picture of the Lo phase provides an explanation for a number of experimental results, which have until now lacked a consistent description in terms of a molecular model:
2H NMR quadropolar splittings
Distinct splittings of the sn-1 and sn-2 DPPC terminal methyls are produced by very ordered hydrocarbon chains aligned parallel to the bilayer normal. This is a distinguishing characteristic for detection of Lo phases. Although the hydrocarbon chains have high order, characteristic of chains with few if any gauche defects, the spectra still indicate rapid reorientation about the bilayer normal of lipids as individual molecules or small clusters. Furthermore, for resolved resonances only one set of quadrupolar splittings per methyl- or methylene group is detected, indicating that the chains explore their entire configurational space on the NMR timescale (correlation times less than 10 μsec). This imposes strong limits on aggregate size.13 In summary, the experimental spectra are in excellent agreement with organization of DPPC in small, highly mobile clusters with hydrocarbon chains packed in a hexagonal lattice and rapid exchange of DPPC molecules.
1H magic-angle spinning NMR
The chain methylene resonances of DPPC in the Ld phase have a typical linewidth of 50–100 Hz. They broaden by a factor of ten in the Lo phase compared to Ld. This is the result of greatly reduced trans-gauche isomerization of chains, consistent with the arrangement of DPPC in mostly trans hexagonally ordered regions.37
NMR diffusion measurements
Mean squared displacements are reduced by roughly a factor of 3 in Lo compared to Ld.38 This is consistent with the slower (but still liquid-like) diffusion observed in Lo, clearly evident in the movie of the Lo phase accompanying Fig. 4.
Small angle X-ray scattering
Bilayer thickness is increased and area per lipid decreased in Lo,39 due to regions of packed DPPC chains.
Small angle neutron scattering
Nanoscale domains with hexagonal order have recently been reported in binary mixtures of DPPC with cholesterol.40
The composition and cholesterol dependence of the Lo phase evoke nanoscale size raft regions in the cell membrane, and yet membrane components that partition to raft regions in cell membranes typically do not partition to the Lo phase in bilayers.6,41 Since many putative raft components have a preference for solvation by cholesterol, within the Lo phase they should partition to the cholesterol rich regions found at the boundaries of the densely packed hexagonal DPPC clusters. In the macroscopic Lo phase observed below Tm for mixtures of DPPC, DOPC, and cholesterol, there is comparatively little cholesterol rich area available to solvate such components. In the resting cell membrane, however, raft regions are known to be quite small,9,10 consistent instead with nanoscale clusters of ordered chains, solvated by a cholesterol rich boundary region. These nanoscale clusters may be driven by T > Tm composition fluctuations, stabilized by immobilized “defects”,42 or they may be a result of reduced line tension from replacing DOPC by POPC.14 Since many small nanoscale clusters have an increased total boundary length relative to a macroscopic phase, components favoring cholesterol would find more such regions accessible. Furthermore, the size and balance of substructure within such regions (and therefore the area available to solvate raft components) in the cell membrane may well be shifted by several mechanisms, including coupling to the cytoskeleton,42–44 proximity to the miscibility transition,11,13,45 or active maintenance by the cell.46,47 Indeed, crosslinking of components that partition into the densely packed substructure provides a natural mechanism to trigger small clusters to coalesce into a macroscopic domain.41 Thus, understanding precisely how the Lo substructure is altered by such mechanisms presents itself as a promising line of inquiry to resolve the connection between lipid bilayer and cell membrane lateral structure.
But why then is cholesterol a necessary component for liquid-liquid coexistence? Beginning with early work on binary mixtures of cholesterol and saturated phospholipids,48–50 it has been hypothesized that the structure and thermodynamics of the Lo phase are driven by direct interactions between cholesterol and saturated lipids. This idea has been elaborated by McConnell and Radhakrishnan in terms of “condensed complexes.”51 The condensed complex model departs from regular solution theory by including a fourth species to model a stoichiometric condensed complex of cholesterol and (in this case) DPPC. An alternate view that proposes a longer range, lattice-like substructure in the Lo phase of binary mixtures containing cholesterol has been proposed by Chong and coworkers, with the lattice composed of condensed complexes of lipid and cholesterol.52
In contrast, the present results indicate that cholesterol disrupts what would otherwise be gel-fluid coexistence, not just by direct interactions with the lipids, but indirectly, because cholesterol prefers an organization that is incommensurate with the local order preferred by DPPC in the Lo phase. Consistent with this idea, Martinez-Seara, et al.20 recently showed that when in binary mixtures with DSPC, cholesterol prefers to interact with other cholesterols in next-nearest neighbor solvation shells at three-fold symmetric orientations, but the acyl chains of DSPC lie at roughly five-fold symmetric positions around cholesterol. Consequently, the rotational symmetry of cholesterol in Lo regions appears to be only partially compatible with hexagonally ordered DPPC chains. One may then view the condensed complex model as a way to incorporate non-mean field density fluctuations into regular solution theory. The present data indicate that these non-mean field fluctuations are not stoichiometric complexes of cholesterol with DPPC, but, rather, DPPC with itself. Indeed, more than 20 years ago Sankaram and Thompson suggested, on the basis of 2H NMR spectra and electron spin resonance measurments of binary mixtures, that the data could be explained not by stoichiometric complexes but by time averaged cholesterol positions.53,54 This hypothesis could be tested by calculations similar to those presented here, replacing cholesterol by other sterols, such as lanosterol. Published 2H NMR55 and quasi-elastic neutron scattering56 studies report sterol-dependent differences in the ordering and dynamics of the lipid chains.
In summary, the Lo phase of a mixture of cholesterol and two lipids is shown to be itself inhomogeneous. Lateral segregation within the Lo phase is observed, with regions of hexagonally packed saturated chains separated by insterstital regions enriched in cholesterol and unsaturated chains. The observed substructure explains existing experimental data, and provides a focus for future efforts aimed at understanding the molecular scale structure of cell membranes.
Supplementary Material
Acknowledgments
Funding Sources
Anton computer time was provided by the National Resource for Biomedical Supercomputing (NRBSC), the Pittsburgh Supercomputing Center (PSC), and the BTRC for Multiscale Modeling of Biological Systems (MMBioS) through Grant P41GM103712-S1 from the National Institutes of Health (NIH). The Anton machine at NRBSC/PSC was generously made available by D.E. Shaw Research. This research was supported in part by the Intramural Research Programs of the National Institute on Alcohol Abuse and Alcoholism, and National Heart, Lung and Blood Institute of the NIH, and utilized the NHLBI LoBoS cluster.
We thank Sarah Veatch for acquisition of the 2H NMR spectra.
Footnotes
Web Enhanced Objects. 3 movies in mpeg format are available.
Supporting Information. 5 Figures. This material is available free of charge via the Internet at http://pubs.acs.org.
References
- 1.Shimshick EJ, McConnell HM. Biochemistry. 1973;12:2351. doi: 10.1021/bi00736a026. [DOI] [PubMed] [Google Scholar]
- 2.Feigenson GW. Annual Review of Biophysics and Biomolecular Structure. 2007;36:63. doi: 10.1146/annurev.biophys.36.040306.132721. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 3.Veatch SL, Keller SL. Biochimica et Biophysica Acta (BBA) - Molecular Cell Research. 2005;1746:172. doi: 10.1016/j.bbamcr.2005.06.010. [DOI] [PubMed] [Google Scholar]
- 4.Bartels T, Lankalapalli RS, Bittman R, Beyer K, Brown MF. Journal of the American Chemical Society. 2008;130:14521. doi: 10.1021/ja801789t. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 5.Hjort Ipsen J, Karlström G, Mourtisen OG, Wennerström H, Zuckermann MJ. Biochimica et Biophysica Acta (BBA) - Biomembranes. 1987;905:162. doi: 10.1016/0005-2736(87)90020-4. [DOI] [PubMed] [Google Scholar]
- 6.Lingwood D, Simons K. Science. 2010;327:46. doi: 10.1126/science.1174621. [DOI] [PubMed] [Google Scholar]
- 7.Pike LJ. Journal of Lipid Research. 2006;47:1597. doi: 10.1194/jlr.E600002-JLR200. [DOI] [PubMed] [Google Scholar]
- 8.Simons K, Ikonen E. Nature. 1997;387:569. doi: 10.1038/42408. [DOI] [PubMed] [Google Scholar]
- 9.Eggeling C, Ringemann C, Medda R, Schwarzmann G, Sandhoff K, Polyakova S, Belov VN, Hein B, von Middendorff C, Schonle A, Hell SW. Nature. 2009;457:1159. doi: 10.1038/nature07596. [DOI] [PubMed] [Google Scholar]
- 10.Sezgin E, Levental I, Grzybek M, Schwarzmann G, Mueller V, Honigmann A, Belov VN, Eggeling C, Coskun Ü, Simons K, Schwille P. Biochimica et Biophysica Acta (BBA) - Biomembranes. 2012;1818:1777. doi: 10.1016/j.bbamem.2012.03.007. [DOI] [PubMed] [Google Scholar]
- 11.Veatch SL, Cicuta P, Sengupta P, Honerkamp-Smith A, Holowka D, Baird B. ACS Chemical Biology. 2008;3:287. doi: 10.1021/cb800012x. [DOI] [PubMed] [Google Scholar]
- 12.Honerkamp-Smith AR, Cicuta P, Collins MD, Veatch SL, den Nijs M, Schick M, Keller SL. Biophysical journal. 2008;95:236. doi: 10.1529/biophysj.107.128421. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13.Veatch SL, Soubias O, Keller SL, Gawrisch K. Proc Nat Acad Sci. 2007;104:17650. doi: 10.1073/pnas.0703513104. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Heberle FA, Petruzielo RS, Pan J, Drazba P, Kučerka N, Standaert RF, Feigenson GW, Katsaras J. Journal of the American Chemical Society. 2013;135:6853. doi: 10.1021/ja3113615. [DOI] [PubMed] [Google Scholar]
- 15.Zhao J, Wu J, Heberle FA, Mills TT, Klawitter P, Huang G, Costanza G, Feigenson GW. Biochimica et Biophysica Acta (BBA) - Biomembranes. 2007;1768:2764. doi: 10.1016/j.bbamem.2007.07.008. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 16.Heberle FA, Wu J, Goh SL, Petruzielo RS, Feigenson GW. Biophysical journal. 2010;99:3309. doi: 10.1016/j.bpj.2010.09.064. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 17.Konyakhina TM, Goh SL, Amazon J, Heberle FA, Wu J, Feigenson GW. Biophysical journal. 2011;101:L8. doi: 10.1016/j.bpj.2011.06.019. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18.Róg T, Pasenkiewicz-Gierula M, Vattulainen I, Karttunen M. Biochimica et Biophysica Acta (BBA) - Biomembranes. 2009;1788:97. doi: 10.1016/j.bbamem.2008.08.022. [DOI] [PubMed] [Google Scholar]
- 19.Khelashvili G, Pabst G, Harries D. The Journal of Physical Chemistry B. 2010;114:7524. doi: 10.1021/jp101889k. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Martinez-Seara H, Róg T, Karttunen M, Vattulainen I, Reigada R. PLoS ONE. 2010;5:e11162. doi: 10.1371/journal.pone.0011162. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Róg T, Pasenkiewicz-Gierula M. Biophysical journal. 2001;81:2190. doi: 10.1016/S0006-3495(01)75867-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 22.Waheed Q, Tjörnhammar R, Edholm O. Biophysical journal. 2012;103:2125. doi: 10.1016/j.bpj.2012.10.014. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 23.Perlmutter JD, Sachs JN. Journal of the American Chemical Society. 2009;131:16362. doi: 10.1021/ja9079258. [DOI] [PubMed] [Google Scholar]
- 24.Pandit SA, Vasudevan S, Chiu SW, Jay Mashl R, Jakobsson E, Scott HL. Biophysical journal. 2004;87:1092. doi: 10.1529/biophysj.104.041939. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25.Niemelä PS, Ollila S, Hyvönen MT, Karttunen M, Vattulainen I. PLoS Comput Biol. 2007;3:e34. doi: 10.1371/journal.pcbi.0030034. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 26.Alameida PFF, Vaz WLC. In: Handbook of Biological Physics. Lipowsky R, Sackman E, editors. Vol. 1. Elsevier Science B.V; Amsterdam: 1995. p. 305. [Google Scholar]
- 27.de Joannis J, Coppock PS, Yin F, Mori M, Zamorano A, Kindt JT. Journal of the American Chemical Society. 2011;133:3625. doi: 10.1021/ja110425s. [DOI] [PubMed] [Google Scholar]
- 28.Shaw DE, Maragakis P, Lindorff-Larsen K, Piana S, Dror RO, Eastwood MP, Bank JA, Jumper JM, Salmon JK, Shan Y, Wriggers W. Science. 2010;330:341. doi: 10.1126/science.1187409. [DOI] [PubMed] [Google Scholar]
- 29.Jo S, Kim T, Iyer VG, Im W. Journal of Computational Chemistry. 2008;29:1859. doi: 10.1002/jcc.20945. [DOI] [PubMed] [Google Scholar]
- 30.Shaw DE, Dror RO, Salmon JK, Grossman JP, Mackenzie KM, Bank JA, Young C, Deneroff MM, Batson B, Bowers KJ, Chow E, Eastwood MP, Ierardi DJ, Klepeis JL, Kuskin JS, Larson RH, Lindorff-Larsen K, Maragakis P, Moraes MA, Piana S, Shan Y, Towles B. Proceedings of the Conference on High Performance Computing Networking, Storage and Analysis. ACM; Portland, Oregon: 2009. p. 1. [Google Scholar]
- 31.Martyna GJ, Tobias DJ, Klein ML. The Journal of Chemical Physics. 1994;101:4177. [Google Scholar]
- 32.Shan Y, Klepeis JL, Eastwood MP, Dror RO, Shaw DE. The Journal of Chemical Physics. 2005;122:054101. doi: 10.1063/1.1839571. [DOI] [PubMed] [Google Scholar]
- 33.Baum LE, Petrie T, Soules G, Weiss N. Ann Math Stat. 1970;41:164. [Google Scholar]
- 34.Welch LR. IEEE Info Theo Soc News. 2003;53:4. [Google Scholar]
- 35.Viterbi AJ. IEEE Trans Info Theo. 1967;13:260. [Google Scholar]
- 36.Lafleur M, Cullis PR, Bloom M. Eur Biophys J. 1990;19:55. doi: 10.1007/BF00185086. [DOI] [PubMed] [Google Scholar]
- 37.Polozov IV, Bezrukov L, Gawrisch K, Zimmerberg J. Nat Chem Biol. 2008;4:248. doi: 10.1038/nchembio.77. [DOI] [PubMed] [Google Scholar]
- 38.Orädd G, Westerman PW, Lindblom G. Biophysical journal. 2005;89:315. doi: 10.1529/biophysj.105.061762. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 39.Mills TT, Tristram-Nagle S, Heberle FA, Morales NF, Zhao J, Wu J, Toombes GES, Nagle JF, Feigenson GW. Biophysical journal. 2008;95:682. doi: 10.1529/biophysj.107.127910. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 40.Armstrong CL, Marquardt D, Dies H, Kučerka N, Yamani Z, Harroun TA, Katsaras J, Shi AC, Rheinstädter MC. PLoS ONE. 2013;8:e66162. doi: 10.1371/journal.pone.0066162. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 41.Hammond AT, Heberle FA, Baumgart T, Holowka D, Baird B, Feigenson GW. Proceedings of the National Academy of Sciences of the United States of America. 2005;102:6320. doi: 10.1073/pnas.0405654102. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 42.Zhao J, Wu J, Veatch SL. Biophysical journal. 2013;104:825. doi: 10.1016/j.bpj.2012.12.047. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 43.Kusumi A, Fujiwara TK, Morone N, Yoshida KJ, Chadda R, Xie M, Kasai RS, Suzuki KGN. Seminars in Cell & Developmental Biology. 2012;23:126. doi: 10.1016/j.semcdb.2012.01.018. [DOI] [PubMed] [Google Scholar]
- 44.Kusumi A, Sako Y. Current Opinion in Cell Biology. 1996;8:566. doi: 10.1016/s0955-0674(96)80036-6. [DOI] [PubMed] [Google Scholar]
- 45.Fan J, Sammalkorpi M, Haataja M. Physical Review Letters. 2010;104:118101. doi: 10.1103/PhysRevLett.104.118101. [DOI] [PubMed] [Google Scholar]
- 46.Fan J, Sammalkorpi M, Haataja M. Physical Review Letters. 2008;100:178102. doi: 10.1103/PhysRevLett.100.178102. [DOI] [PubMed] [Google Scholar]
- 47.Gowrishankar K, Ghosh S, Saha S, CR, Mayor S, Rao M. Cell. 2012;149:1353. doi: 10.1016/j.cell.2012.05.008. [DOI] [PubMed] [Google Scholar]
- 48.Gershfeld NL. Biophysical journal. 1978;22:469. doi: 10.1016/S0006-3495(78)85500-3. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 49.Hinz HJ, Sturtevant JM. Journal of Biological Chemistry. 1972;247:3697. [PubMed] [Google Scholar]
- 50.Presti FT, Pace RJ, Chan SI. Biochemistry. 1982;21:3831. doi: 10.1021/bi00259a017. [DOI] [PubMed] [Google Scholar]
- 51.Radhakrishnan A, McConnell H. Proceedings of the National Academy of Sciences of the United States of America. 2005;102:12662. doi: 10.1073/pnas.0506043102. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 52.Sugár IP, Chong PLG. Journal of the American Chemical Society. 2011;134:1164. doi: 10.1021/ja2092322. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 53.Sankaram MB, Thompson TE. Biochemistry. 1990;29:10676. doi: 10.1021/bi00499a015. [DOI] [PubMed] [Google Scholar]
- 54.Sankaram MB, Thompson TE. Proceedings of the National Academy of Sciences. 1991;88:8686. doi: 10.1073/pnas.88.19.8686. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 55.Martinez GV, Dykstra EM, Lope-Piedrafita S, Brown MF. Langmuir. 2004;20:1043. doi: 10.1021/la036063n. [DOI] [PubMed] [Google Scholar]
- 56.Endress E, Heller H, Casalta H, Brown MF, Bayerl TM. Biochemistry. 2002;41:13078. doi: 10.1021/bi0201670. [DOI] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.