Abstract
Regulation of cell growth and proliferation has a fundamental role in animal and plant development and in the progression of cancer. In the context of development, it is important to understand the mechanisms that coordinate growth and patterning of tissues. Imaginal discs, which are larval precursors of fly limbs and organs, have provided much of what we currently know about these processes. Here, we consider the mechanism that is responsible for the observed uniformity of growth in wing imaginal discs, which persists in the presence of gradients in growth inducing morphogens in spite of the stochastic nature of cell division. The phenomenon of “cell competition,” which manifests in apoptosis of slower-growing cells in the vicinity of faster growing tissue, suggests that uniform growth is not a default state but a result of active regulation. How can a patch of tissue compare its growth rate with that of its surroundings? A possible way is furnished by mechanical interactions. To demonstrate this mechanism, we formulate a mathematical model of nonuniform growth in a layer of tissue and examine its mechanical implications. We show that a clone growing faster or slower than the surrounding tissue is subject to mechanical stress, and we propose that dependence of the rate of cell division on local stress could provide an “integral-feedback” mechanism stabilizing uniform growth. The proposed mechanism of growth control is not specific to imaginal disc growth and could be of general relevance. Several experimental tests of the proposed mechanism are suggested.
Keywords: Drosophila melanogaster, imaginal disc, mechanics, stress
Understanding the principles and mechanisms involved in animal and plant development remains an outstanding challenge for modern biology (1, 2). Among the fundamental problems is the problem of understanding how organisms (or organs and body parts) coordinate their growth with internal patterning to achieve correct size and proportions (3–5). A fruit fly, Drosophila melanogaster, has been an invaluable model of development in general and organogenesis in particular (6, 7). Below, we focus on the question of growth control (8, 9) in the context of a wing imaginal disc (7), which is the larval precursor of the adult wing. We point out that nonuniform growth in a layer of cells that adhere to each other (as is the case for imaginal discs) leads to accumulation of mechanical stress. This stress may provide cells with a feedback signal, regulating cell division and insuring stable and uniform growth of tissue. To describe this process quantitatively, we formulate and analyze a mathematical model of mechanical deformation in growing tissue. We show that the proposed mechanical feedback model could naturally explain certain salient features of growth in imaginal discs, such as “cell competition” (6, 10, 11), and suggest experiments that would directly test the model.
The possible role of mechanical interactions in growth has been suggested, most explicitly in the context of plant development (12–15). However, mechanics has been demonstrated to have a role in regulation of cellular processes in animals as well. Specialized mechanosensory cells use mechanically gated ion channels as sensors (16, 17). Compressive and tensile stresses are known to be important in muscle and bone tissues and tension-sensitive signaling has been reported in tissue cultures (18–20). Also suggestive of mechanical interactions are the observations of the effects of geometric constraints on the growth of cultured endothelial cells (21). Focal adhesion complexes and adherens junctions (through which cells interact with the substrate and each other, respectively) have been argued as the likely loci of mechanosensation (22, 23). There is evidence for mechanical stress-induced ectopic expression of genes in Drosophila embryo (24), which appears to be mediated by Armadillo, a protein that in one of its roles serves as a scaffold for the assembly of cell-adhesion junctions with cortical actin (25, 26). And most recently, a link between mechanical deformation, actin cytoskeleton morphology, and transcription factors [Mal-D and serum response factor (SRF)] has been demonstrated in the migrating border cells during Drosophila oogenesis (27). On the theory side, modeling of tissue mechanics during development has been discussed before in the context of convergent extension (28) and in the context of cell sorting and differential adhesion (29–31).
Wing imaginal disc derives from a patch of ≈50 embryonic cells, which by the time of growth arrest occurring at pupation, will multiply by means of cell division to ≈5 × 104 (7). Patterning of imaginal discs proceeds concurrently with growth, although at that stage it is evident only on the level of gene expression. The pattern of expression of certain key transcription factors is organized relative to antero-posterior and dorso-ventral axes (32–34) and is generated in response to gradients in concentration of morphogens (35): Decapentaplegic (Dpp) (32, 33) for antero–posterior and Wingless (34, 36) for the dorso–ventral axes. Notably, both morphogens are also required for tissue growth (37, 38).
A very appealing proposal for a mechanism of size determination exists (3, 35, 39); the idea is that growth is controlled by a gradient of “positional values.” Positional value is a yet unidentified property of the cell, which becomes a fixed attribute of the cell upon the birth of the cell, with its value interpolating those of neighboring cells (3, 35, 39). As tissue grows, new cells intercalate between cells, gradually decreasing positional value differential between neighboring cells until it falls below a threshold and growth stops (39). The most explicit scenario of this type (3) suggests that the role of positional value is played by the morphogen concentration itself and, hence, that growth is driven by morphogen gradient. This idea is appealing because it offers an explicit link between growth and patterning of gene expression. However, this scenario is contradicted‡ by experimental observation that Dpp, and not its gradient, drives cell proliferation (32, 40).
The observation that growth is driven directly by morphogen level leads to another puzzle. The rate of proliferation within the wing pouch of the imaginal disc is observed to be on average uniform (9), yet morphogen level is not. One immediate interpretation would be that the rate of cell division is constant, provided that morphogens (Dpp and Wingless) are locally above a certain threshold (5). Yet, the threshold model is not likely to be the explanation because ectopic expression of Dpp (40) can locally drive cell overgrowth, which means that growth rate is not saturated and insensitive to the increase in Dpp level. Furthermore, there is a body of evidence suggesting an interaction between patches of tissue that proliferate at different rates. This phenomenon is known as cell competition. Clones with compromised growth rate (e.g., MinuteA) are eliminated from the WT background but develop normally if set within similarly compromised (e.g., MinuteB) background tissue (6, 11, 41). On the other hand, clones with enhanced growth rate (Minite+) can take over the compartment made up of WT cells. It appears, that in this process, slower-growing WT cells near the edge of a faster growing mutant clone are eliminated through apoptosis allowing the mutant clone to invade and expand at the expense of normal WT cells (41). Similar behavior is observed with mutant clones expressing constitutively activated Dpp receptor (tkv*) (42) and clones overexpressing myc (10, 43, 44). Understanding possible mechanisms by which patches of tissue communicate and compare their growth rates is a major open question that we address below.
The disc is composed of a single 2D layer of epithelial cells (7), which are joined together by adherens junctions (AJs) (25, 45) located near the apical surface of the cells. AJs involve cadherins, which are the transmembrane proteins that bind apposing cell surfaces while simultaneously joining the cortical actin filaments on the intracellular side. The result is a tissue-wide elastic network. It is believed that there is little or no relative cell motion beyond local rearrangements associated with cell division. The cells are strongly elongated in the direction transverse to the layer, and the thickness of the cell layer is not quite uniform throughout the disc (it is thicker in the center) and changes over the 120 h of disc development within larva.
Mechanics of Inhomogeneous Growth
Let us consider mechanical implications of nonuniform growth in the imaginal disc. Relegating the mathematical formulation and the analysis of the problem to Appendix and Supporting Appendix, which is published as supporting information on the PNAS web site, here we only describe the model and key results. Because no significant global cell rearrangement is observed within the imaginal disk layer, we assume that it has mechanical properties of a solid (rather than a fluid) and, thus, has certain rigidity to shear (46), at least on the time scale of cell cycle. On length scales larger than the size of individual cells, mechanical equilibrium of the tissue is then governed by minimization of elastic energy appropriate to a 2D elastic medium. This consideration allows one to determine the displacement of cells away from their initial positions that result from cell growth and proliferation that occurs during a Δt increment of time. We define local rate of tissue growth, γ(r, t), as the relative rate of increase in tissue area, which in the absence of mechanical constraints would lead to an increase in small “clone” area, A, given by dA/dt = γ(r, t)A. Uniform growth of tissue generates a uniform local expansion without creating any local compression or dilation stresses. However, if one imagines a compact patch of tissue growing faster than its surroundings, it is intuitively evident that this patch of tissue will be compressed and that the surrounding tissue will be strained. In Appendix, we derive the explicit relation between excess pressure, p(r, t), within a small tissue (Fig. 1) patch (at position r, at time t) and the history of its growth relative to that of the tissue.§ The rate of local pressure increase is proportional to the difference between the local growth rate and the average growth rate, 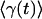 , of surrounding tissue and scales with the shear-rigidity modulus μ (and the bulk modulus K, see Appendix and ref. 46):
, of surrounding tissue and scales with the shear-rigidity modulus μ (and the bulk modulus K, see Appendix and ref. 46):
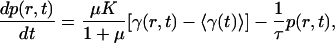 |
[1] |
so that the state of compression (or dilation) of a given patch of tissue (e.g., a small clone) is determined by the integral over the past history of its growth rate compared with that of the tissue on average. The effect would disappear in a tissue without shear rigidity (i.e., μ = 0) and, thus, depends critically on cells adhering to each other and their inability to flow in response to pressure. However, slow rearrangement is represented in Eq. 1 by a relaxation term, –τ-1p(t), which effectively limits the “memory” of growth to time of order, τ, characteristic of the rearrangement process. Even if negligible at low stress, relaxation could become fast enough to be important as soon as the stress exceeds certain critical level and tissue yields to cell rearrangement resulting in plastic flow (46). This effect can also be captured by a relaxation term, with τ depending on pressure. Note that, in deriving Eq. 1, we assumed that the boundaries are so far away that their effect is negligible. However, it can be readily generalized to include the effect of boundary conditions.¶
Fig. 1.
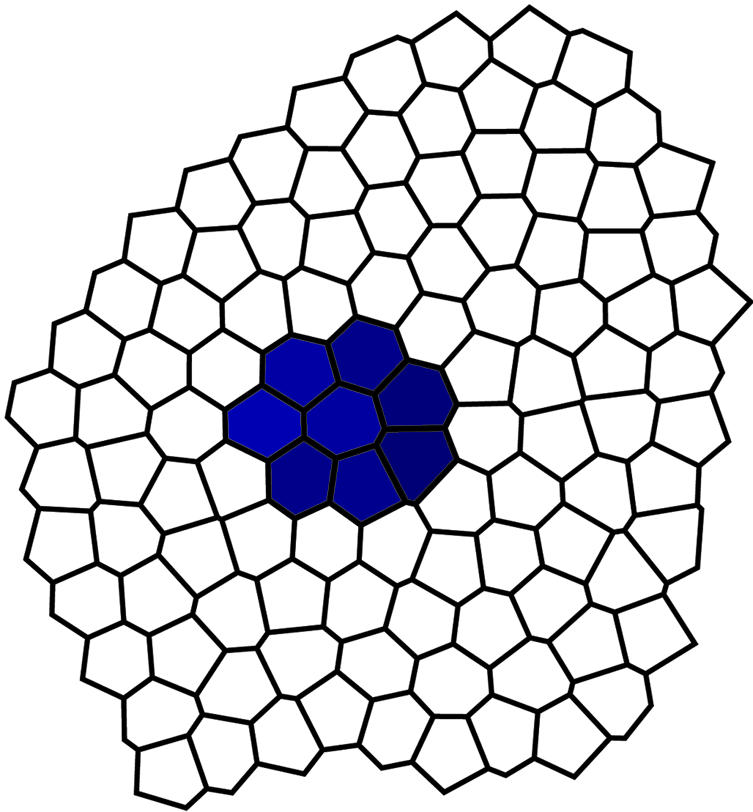
A clone of cells carrying a certain somatic mutation forms a patch of a 2D tissue layer with different rate of cell growth and proliferation.
Compression of cells within the layer can be at least partially relieved by the buckling of cell layer out of the plane (Fig. 2). Such an instability occurs as soon as compression over given area exceeds certain threshold (see Supporting Appendix) and is likely to be used by organisms to form 3D structures. In the context of imaginal discs, buckling occurs sometimes for overgrowing mutant clones (40). However, it is observed neither in WT nor in most mutant cases without compromised apoptosis. We interpret this fact as indirect evidence that cells normally possess a mechanism that can regulate local tissue growth rate γ(r, t) to avoid excessive local compression and buckling. Actually, tissue growth regulation may be broken down into three distinct components, (i) regulation of cell division rate, (ii) regulation of cell growth rate, and (iii) activation of apoptosis. The γ(r, t) variable represents their combined effect (as explained in Supporting Appendix).
Fig. 2.
Schematic representation of a crosssection of a buckled region of a cell layer.
Mechanical Feedback Mechanism
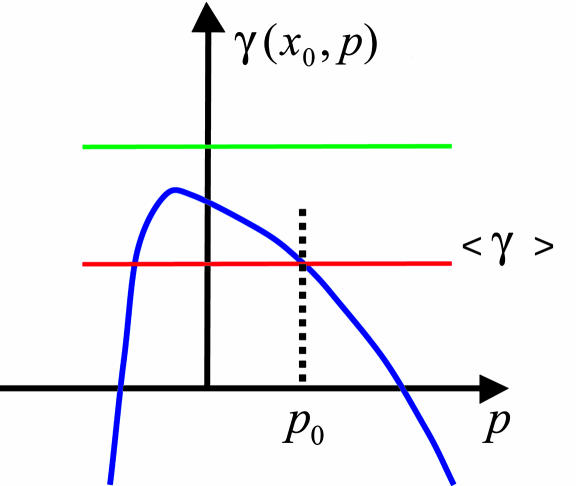
Any mechanism coordinating growth within tissue would require an input signal containing information that allows each cell to compare its rate of growth and division with that of the surrounding tissue. Mechanical interactions are very interesting in this respect, because they immediately provide information that is necessary for a regulatory feedback mechanism.∥ For example, suppose that local tissue growth rate γ(r, t, p(r, t)) depends explicitly on the degree of local compression (or stretching) of the tissue as quantified by p(r, t) and this dependence has a form as shown in Fig. 3, which assumes only that (i) growth rate depends on pressure and (ii) large mechanical stress suppresses growth and triggers apoptosis (which corresponds to γ < 0). Given the dependence of pressure on the growth-rate differential described by Eq. 1, we show in Fig. 3 how mechanical feedback could robustly lead either to the equilibration of growth rates or elimination of growth-impaired clones.
Fig. 3.
Pressure dependence of local tissue growth rate (the growth curve) and the feedback effect scenarios. Blue curve represents an assumed growth curve for a mutant clone. Negative γ represents apoptosis. In the case in which background growth rate (red line) is slower than the rate of clone growth, the growth-rate differential leads to the increase of local pressure within the clone until it reaches p0, at which the rates of growth for the clone and background equalize. For the case of growth-impaired clones, the rate of background growth (represented by the green line) can be higher than the maximal possible rate of clone growth. The growth-rate differential then leads to a decrease in local pressure and if blue and green curves do not intersect, the rate of growth in the clone cannot catch up with that of the background leading to continuing build-up of negative pressure (i.e., tension). Excessive tension in the tissue and the consequent distortion of cells may trigger apoptosis. This scenario could explain elimination of slow-growing clones by cell competition (6, 11).
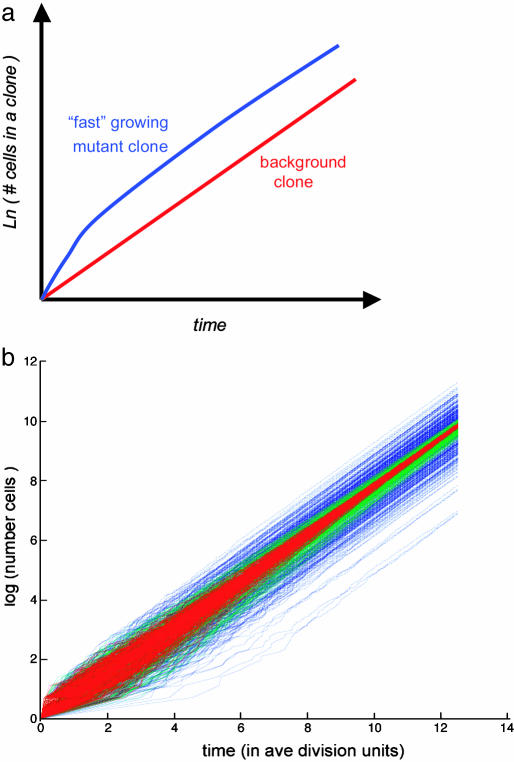
According to the feedback scenario shown in Fig. 3, a faster-growing clone would be larger than the WT clone of the same “age,” but the difference would not be increasing exponentially in time, as might be expected on the basis of different growth rates. Instead, the mutant clone would be larger than WT by a fixed factor (see Fig. 4a), the magnitude of which depends on the strength of the feedback (i.e., sensitivity of growth rate to pressure given by the blue curve in Fig. 3) and shear rigidity of tissue, μ. For a slow-growing clone, the analysis shown in Fig. 3 predicts inevitable death by apoptosis.
Fig. 4.
Growth of clone size (defined as the number of cells in the clone) with time. (a) Expected time course of clone size. The average number of cells in a clone grows exponentially. Red indicates expected growth of a background clone. Blue indicates expected growth of a mutant clone with accelerated rate of proliferation in the presence of growth feedback. Initial rate of growth is faster than that of a background clone. At later stages, the rate of growth is reduced to match that of the background, resulting in a ratio of mutant to background clone sizes (the overgrowth ratio) independent of time. (b) Simulated time course of clone size for a stochastic cell division process modeled by two independent sequential random Poisson processes with equal rates. Blue lines represent different realizations of the random process. Note that most of the variance of the clone size distribution is acquired at early times when the clones have a small number of cells, but the variance of the size distribution does not decrease with time. Green and red lines represent realizations of the growth process with a linear integral feedback of increasing strength. Integral feedback causes the clones that have attained larger than average size early on to grow more slowly. This effect results in a decrease of variance of with time.
The regulatory feedback mechanism proposed above involves the integral of the difference between local and average rates of growth naturally furnished by mechanical stress (Eq. 1). This mode of regulation, which is called “integral feedback” in control theory (47), is the method of choice in engineering design because of its stability properties and insensitivity to parameters (such as details of the pressure dependence of γ as shown in Fig. 3). Stress relaxation due to cell rearrangement and plastic flow (τ-1 term in Eq. 1), which we have so far neglected in the discussion of the feedback, limits the “memory” of integration making the adaptation of growth rate imperfect. Similar limitation typically applies in the engineering implementation of integral feedback but, provided that relaxation is sufficiently slow, it does not significantly compromise feedback function.
Nonautonomous Effects
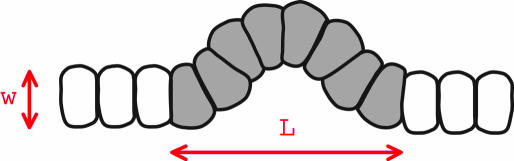
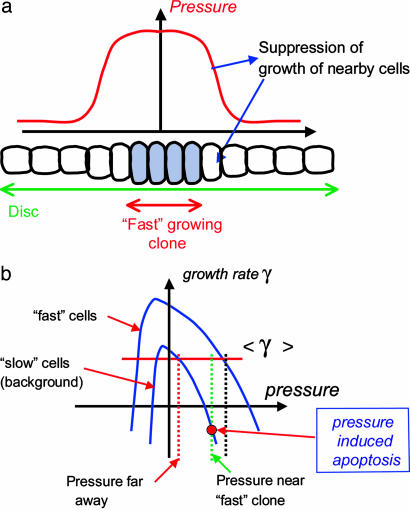
We have discussed mechanical feedback on the basis of the quasi-local relation between pressure and the growth rate given by Eq. 1. The latter was derived for idealized 2D elasticity. It is more appropriate to treat epithelial cell layer as an elastic layer of finite thickness. Analysis of in-plane deformations induced by nonuniform growth in this case will modify Eq. 1 by introducing the following term on the left side: w2▿2(d/dt)p(r, t) with w being a characteristic length scale comparable with cell size.** The effect of this term is to smoothen out any spatial variation of the pressure induced by nonuniform growth, with the characteristic length scale for pressure averaging provided by w (Fig. 5a).
Fig. 5.
Nonautonomous effects of nonuniform growth and a possible scenario for cell-competition phenomenon. (a) Accelerated growth within an isolated clone causes compression of nearby tissue (and a distortion of cells decreasing in inverse proportion to the distance). (b) Growth curves for fast growing mutant clone cells (upper blue curve) and the slower-growing background tissue (lower blue curve). Red line represents the average growth rate of the background. Mutant clone overgrows until the resulting compression (black dashed line) brings its growth rate down to that of the background. Level of compression of WT tissue neighboring the clone (green dashed line) may be sufficiently high to trigger the apoptosis of the slower-growing WT cells near the clone.
One consequence of the nonlocality of the induced stress is that if a faster growing mutant clone has generated high level of compression, it will be felt nonautonomously by nearby (i.e., within w distance from the border) WT cells. If the pressure is sufficiently high, as shown in Fig. 5b, this effect can cause apoptosis in the WT cells surrounding overgrowing clones. Death of surrounding cells would partially relieve the pressure on the clone, allowing additional growth of the mutant clone at the expense of WT tissue. In the case of growth-compromised clones, apoptosis appeared as an inescapable consequence of the inability of the growth compromised cell to catch up with the surrounding tissue (Fig. 3). By contrast, in the present case, the fate of the cells surrounding an overgrowing clone depends on the sensitivity of these cells and those in the overgrowing clone to pressure [i.e., apoptosis is predicted only for the case in which the stabilizing pressure p0 (see Fig. 5b) exceeds the threshold of apoptosis for the surrounding tissue]. Thus, the mechanical feedback scenario may provide an explanation for the observed cell-competition phenomena (6, 11, 41, 43) described in the Introduction.
Because mechanical interactions are nonlocal, interaction effects between nonadjacent clones and clones and compartment boundaries are expected. Mechanical stresses could also have an important role in determining the shape of clones by slowing down division of distorted cells and, perhaps, orienting mitotic spindles along the stretching axis.
Effect of Feedback and Possible Experimental Tests
For experimental verification, we could decompose the mechanism proposed above into two hypotheses, (i) existence of feedback regulation of local growth rate, and (ii) mechanical origin of the feedback signal. It would be interesting to examine quantitatively as a function of time the extent of overgrowth resulting from mutations that accelerate cell cycle. Our model predicts that the ratio of average mutant clone size (or cell number) to that of a WT clone of same age would initially increase but then saturate at a constant value (see Fig. 4a). This behavior is in contrast to continued exponential growth of the size ratio with time, which would be expected in the absence feedback. Observation of saturation of this “overgrowth ratio” would be a strong indication of feedback regulation of growth. However, it would provide no clue to the origin of the feedback signal. The first step in establishing the role of mechanical interactions would be to document presence of cell deformation induced by local tissue overgrowth. It would be interesting to obtain high-quality images (apical surface and layer crosssection) of cells surrounding a small clone containing mutation that increases cell size. The presence or absence of deformation would respectively confirm or falsify the crucial assumption that adhesive interaction constrains the cells and prevents the flow that could relieve local pressure. If this assumption were confirmed, the next step would be to investigate the effect of weakening cell adhesion throughout the tissue. In the latter case, the mechanical feedback mechanism would predict an increase in the overgrowth ratio.
Feedback regulation of cell proliferation may have a role not only in controlling the overgrowth of mutant clones but also in reducing the effect of temporal fluctuations in cell division. Indeed, cell division occurs randomly throughout the disk tissue (with apparently random orientation of mitotic spindles) (9). The resulting distribution in the number of cells in WT clones of any given age is very broad (48) and must be considered on a logarithmic scale. The latter is to be expected for a multiplicative random process such as cell proliferation. We find that distribution of cell numbers in relatively “young” clones (48) is consistent with division being governed by a two-step Poisson process (i.e., a random process involving two consecutive random steps occurring at the same average rate). As shown in Fig. 4b, the variance of the logarithm of the number of cells in a clone behaves differently with and without feedback. In the latter case, variance saturates, whereas in the former case, it would decrease with time after the initial rise.
Discussion
In the context of mechanical effects, growth-affecting mutations act by means of two parameters, (i) rate of cell mass acquisition, or alternatively, the rate, α, with which cell area would grow (in the in the absence of mechanical constraint); and (ii) average rate of cell division, β. Together, these two parameters determine the average area of a cell, α/β, and the average density of cells, n = β/α. The rate of tissue growth, which featured in tissue growth mechanics discussed above, is given by γ(r) = α(r)n(r) (see Supporting Appendix for details), where we explicitly allow for possible positional dependence by means of r.
Known mutants are often classified as “compensated” (e.g., cyclin D) or “noncompensated” (e.g., PI3K, Myc, RBF, and E2F; ref. 10) depending on whether they coordinate cell growth with division to maintain constant cell size (i.e., constant α/β). From the point of view of mechanical interactions, we must instead consider effect of mutation on γ. We observe that mutations which affect only cell growth rate α (without a change in β) have only a transient effect on γ (within a cell-cycle time of induction) because, in the steady state, the effect of α on average tissue growth rate would be compensated by the change in local cell density, such that γ = αn = β. Only mutations affecting the cell-division rate β affect the tissue-growth rate γ in a steady state. However, α-type mutants [which, like Myc (10), affect cell size but not rate of cell division] make clones that, while growing at same exponential rate as WT, are still larger (or smaller) than WT by a constant factor. In the absence of any feedback, this factor would be the ratio of mutant to WT single-cell areas. The excess size of such clones would still generate excess local pressure (which, according to Eq. 1, is the integral of growth-rate difference) and reduce the rate of proliferation of cells within and in the vicinity of such clones. If the α-type mutation causes reduction of cell and clone size, our model predicts that the consequent tensile stress within the clone would reduce its growth rate, causing further increase in tensile stress and eventual elimination of the clone by apoptosis. We expect a major phenotypic difference between mutants to arise from different form of “growth curves” γ(p) for different genotypes. The sensitivity of γ to stress would determine the extent of overgrowth and the extent of apoptosis of surrounding tissue. Dependence of growth rate on stress and resulting cell distortion could account for some of the features of cell competition, as explained above. However, cell competition is likely to be a complex phenomenon, caused not only by the differential growth rate but also by difference in cell identity (11, 43, 49, 50).
The discussion of the mechanical effect of nonuniform growth was based on two fundamental approximations, that (i) cells are not free to move within the tissue on the time scale of cell division and (ii) the epithelial tissue layer remains flat. If the cells were free to move, all stresses induced by nonuniform growth could be relieved and the mechanism described above would not operate. However, if cell rearrangement (and plastic flow) were slow, all of the above discussion would apply with the sole modification that mechanical stresses would have a finite “memory” and over-pressure would saturate at a constant value. Here, our discussion was limited to linear elasticity. A more detailed analysis including nonlinear effects can be carried out with the help of numerical simulations. To address the second issue, we note that buckling of a 2D sheet is an instability that requires a finite threshold for local strain. Buckling does not occur in the wing pouch unless normal growth regulation, which preserves tissue planarity, is disrupted. However, reproducible folding in the late stages of normal imaginal disk growth could be naturally attributed to the mechanical effects due to nonuniform growth and spatial modulation of the adhesive properties of the tissue (and, hence, of its mechanical rigidity, μ). It would be interesting to investigate further the role of nonuniform growth in generating 3D tissue structures in animal and plant development (12–14, 51, 52). An interesting extension of the present model would explore the possibility that mechanical feedback effect depends not only on the (2D) pressure but also on the inplane deviatoric stress (46). It is quite possible that the process of cell division is not in itself isotropic; e.g., the direction of mitotic spindle (and the daughter cell axis) may actually be correlated with local in-plane stress axis (15).
It is important to emphasize that the main advantage of mechanical feedback in growth regulation is that it would be driven directly by the nonuniformity of the growth rate. In contrast, any chemical signaling mechanism would require “finetuning” (of messenger secretion and signal transduction) for the signal to correctly represent the difference of the growth rate of a cell with that of its neighbors. For example, it has been proposed that cell competition operates through competition for Dpp (53). Although it is true that rapid uptake of Dpp by any cell would have a nonautonomous effect, it would not directly depend on the growth-rate differential but, rather, on the difference of cell identity (e.g., their genetic make up).
Ultimately, one would want to identify the molecular mechanism underlying possible effect of mechanical stress on cell growth and proliferation. An intriguing possibility discussed here focuses on Armadillo (β-catenin), which is known to play central role in the transduction of Wingless (Wnt) signal, which is known to regulate tissue growth and, at the same time, serve as an adaptor in the assembly of the transcellular E-cadherin/actin network (25, 26, 54, 55). Armadillo was implicated also in the transduction of stress effect on expression of Twist in fly embryo (24). Alternatively, transcription factor MalD [and serum response factor (SRF)] (27) has been implicated in mechanical stress-driven response in motile cells and could be a plausible mediator of stress effects in the present context as well. Another recently proposed mechanism of mechanotransduction (56) involves modulation of the growth factor concentration in the intercellular space resulting from compression of the latter by mechanical pressure. Clearly, new experiments are needed to establish the presence of stress induced interaction and its molecular mechanism in growing epithelial tissue.
Last, we note that mechanical feedback on tissue growth, if present, would have a role in the progression of cancer.
Supplementary Material
Acknowledgments
I thank N. Baker, S. Cohen, C. Desplan, K. Irvine, L. Johnston, A. Levine, A. Ruckenstein, P. Rorth, A. Sengupta, A. Teleman, and L. Wolpert for stimulating discussions. The cell array in Fig. 1 was generated by a numerical simulation of H. Roualt (personal communication). This work was supported by National Institutes of Health Grant GM67794.
Appendix: Mechanical Stress in Nonuniformly Growing Tissue
On length scales that are large compared with cell size and cell-layer thickness, the deformation of the tissue may be described in a continuum approximation. Let vector Δua(r) denote the incremental displacement of a tissue patch (initially at position r) after a small time interval Δt. It is determined by minimization of the elastic strain energy given by the following:
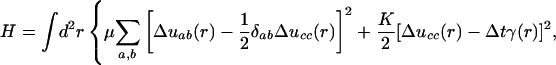 |
[A-1] |
where
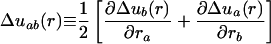 |
is the strain tensor quantifying spatial nonuniformity of Δu(r) (and we are using the convention that repeated indices are summed). K is the bulk modulus and μ is the shear rigidity (47). Tissue displacement is driven by the action of nonuniform tissue growth with a spatially nonuniform rate γ(r) acting over the time interval Δt. This elastic energy minimization is appropriate as long as growth is slow compared with elastic response. Elastic energy, H, is minimized by displacement vector obeying:
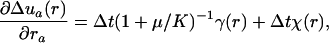 |
[A-2] |
with a scalar function χ(r) satisfying ∂2aχ = 0 and determined by the boundary conditions. Eq. A-2 is analogous to electrostatics, with the right side acting like charge density and Δua playing the role of electrostatic field. Having determined incremental displacement Δua, one can compute the incremental change in pressure:
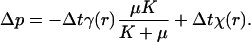 |
[A-3] |
Because for uniform growth with free boundary conditions tissue displacement corresponding to uniform dilation Δua(r) = Δtγ ra/2 accommodates local increase in area without generating any stress we have, for nonuniform growth with free boundaries:
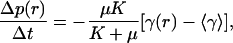 |
[A-4] |
where 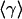 is the rate of growth averaged over the tissue. The first term explicitly shows that local pressure is driven by the difference between local growth rate and the average growth rate and that it arises through the action of shear rigidity μ. A more detailed derivation and discussion is given in Supporting Appendix.
is the rate of growth averaged over the tissue. The first term explicitly shows that local pressure is driven by the difference between local growth rate and the average growth rate and that it arises through the action of shear rigidity μ. A more detailed derivation and discussion is given in Supporting Appendix.
This paper was submitted directly (Track II) to the PNAS office.
Abbreviation: Dpp, Decapentaplegic.
Footnotes
Another problem involves the proposed notion that morphogen gradient decreases in inverse proportion with the size of the tissue. The latter behavior does not follow from the laws of diffusion without an additional assumption that the rate of Dpp secretion at the antero-posterior boundary itself decreases in inverse proportion with the size.
For simplicity of presentation, we discuss here 2D pressure in an idealized 2D tissue. In reality, this 2D pressure would be the uniaxial stress in the cell layer corresponding to the modulation of layer thickness and cell (apical) area.
The right side of Eq. 1 vanishes for uniform γ, which thus yields stress-less growth. More generally, stress-less growth can be produced by a special class of nonuniform growth patterns: those with γ satisfying Δ2γ = 0 (see Supporting Appendix).
One can imagine an alternative mechanism in which cells communicate their proliferation rate (or their local density) to their neighbors by means of a chemical messenger (or cell contact interaction). However, to compare their own growth rate with that of the surrounding tissue, cells would have to correctly calibrate the received signal by fine tuning signal-transduction parameters.
This characteristic length does not necessarily correspond to the thickness of the disc, because the elasticity of the tissue is likely to reside in a much thinner apical cytoskeleton layer.
References
- 1.Sean, B. Carroll, S. W. & Grenier, J. (2000) From DNA to Diversity: Molecular Genetics and the Evolution of Animal Design (Blackwell Science, Oxford).
- 2.Gerhart, J. & Kirschner, M. (1997) Cells, Embryos, and Evolution: Towards a Cellular and Developmental Understanding of Phenotypic Variation and Evolutionary Adaptability (Balckwell Science, Oxford).
- 3.Day, S. J. & Lawrence, P. A. (2000) Development (Cambridge, U.K.) 127, 2977-2987. [DOI] [PubMed] [Google Scholar]
- 4.Conlon, I. & Raff, M. (1999) Cell 96, 235-244. [DOI] [PubMed] [Google Scholar]
- 5.Serrano, N. & O'Farrell, P. H. (1997) Curr. Biol. 7, R186-R195. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 6.Lawrence, P. (1992) The Making of a Fly (Blackwell Science, Oxford).
- 7.Held, L. I. J. (2002) Imaginal Discs: Thr Genetic and Cellular Logic of Pattern Formation (Cambridge Univ. Press, Cambridge, U.K.).
- 8.Hipfner, D. R. & Cohen, S. M. (2004) Nat. Rev. Mol. Cell Biol. 5, 805-815. [DOI] [PubMed] [Google Scholar]
- 9.Milan, M., Campuzano, S. & Garcia-Bellido, A. (1996) Proc. Natl. Acad. Sci. USA 93, 640-645. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 10.Johnston, L. A. & Gallant, P. (2002) BioEssays 24, 54-64. [DOI] [PubMed] [Google Scholar]
- 11.Morata, G. & Ripoll, P. (1975) Dev. Biol. 42, 211-221. [DOI] [PubMed] [Google Scholar]
- 12.Rolland-Lagan, A. G., Bangham, J. A. & Coen, E. (2003) Nature 422, 161-163. [DOI] [PubMed] [Google Scholar]
- 13.Coen, E., Rolland-Lagan, A. G., Matthews, M., Bangham, J. A. & Prusinkiewicz, P. (2004) Proc. Natl. Acad. Sci. USA 101, 4728-4735. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Bohn, S., Andreotti, B., Douady, S., Munzinger, J. & Couder, Y. (2002) Phys. Rev. E Stat. Phys. Plasmas Fluids Relat. Interdiscip. Top. 65, 061914. [DOI] [PubMed] [Google Scholar]
- 15.Lynch, T. M. & Lintilhac, P. M. (1997) Dev. Biol. 181, 246-256. [DOI] [PubMed] [Google Scholar]
- 16.Hamill, O. P. & Martinac, B. (2001) Physiol. Rev. 81, 685-740. [DOI] [PubMed] [Google Scholar]
- 17.Gillespie, P. G. & Walker, R. G. (2001) Nature 413, 194-202. [DOI] [PubMed] [Google Scholar]
- 18.Epstein, N. D. & Davis, J. S. (2003) Cell 112, 147-150. [DOI] [PubMed] [Google Scholar]
- 19.Suter, D. M. & Forscher, P. (2001) J. Cell Biol. 155, 427-438. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Riveline, D., Zamir, E., Balaban, N. Q., Schwarz, U. S., Ishizaki, T., Narumiya, S., Kam, Z., Geiger, B. & Bershadsky, A. D. (2001) J. Cell Biol. 153, 1175-1186. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Dike, L. E., Chen, C. S., Mrksich, M., Tien, J., Whitesides, G. M. & Ingber, D. E. (1999) In Vitro Cell. Dev. Biol. Anim. 35, 441-448. [DOI] [PubMed] [Google Scholar]
- 22.Ko, K. S. & McCulloch, C. A. (2001) Biochem. Biophys. Res. Commun. 285, 1077-1083. [DOI] [PubMed] [Google Scholar]
- 23.Bershadsky, A. D., Balaban, N. Q. & Geiger, B. (2003) Annu. Rev. Cell Dev. Biol. 19, 677-695. [DOI] [PubMed] [Google Scholar]
- 24.Farge, E. (2003) Curr. Biol. 13, 1365-1377. [DOI] [PubMed] [Google Scholar]
- 25.Steinberg, M. S. & McNutt, P. M. (1999) Curr. Opin. Cell Biol. 11, 554-560. [DOI] [PubMed] [Google Scholar]
- 26.Cox, R. T., Kirkpatrick, C. & Peifer, M. (1996) J. Cell Biol. 134, 133-148. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 27.Somogyi, K. & Rorth, P. (2004) Dev. Cell 7, 85-93. [DOI] [PubMed] [Google Scholar]
- 28.Odell, G., Oster, G., Burnside, B. & Alberch, P. (1980) J. Math. Biol. 9, 291-295. [DOI] [PubMed] [Google Scholar]
- 29.Forgacs, G., Foty, R. A., Shafrir, Y. & Steinberg, M. S. (1998) Biophys. J. 74, 2227-2234. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 30.Beysens, D. A., Forgacs, G. & Glazier, J. A. (2000) Proc. Natl. Acad. Sci. USA 97, 9467-9471. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 31.Steinberg, M. S. (1996) Dev. Biol. 180, 377-388. [DOI] [PubMed] [Google Scholar]
- 32.Nellen, D., Burke, R., Struhl, G. & Basler, K. (1996) Cell 85, 357-368. [DOI] [PubMed] [Google Scholar]
- 33.Lecuit, T., Brook, W. J., Ng, M., Calleja, M., Sun, H. & Cohen, S. M. (1996) Nature 381, 387-393. [DOI] [PubMed] [Google Scholar]
- 34.Neumann, C. J. & Cohen, S. M. (1997) Development (Cambridge, U.K.) 124, 871-880. [DOI] [PubMed] [Google Scholar]
- 35.Wolpert, L. (1989) Development (Cambridge, U.K.) 107, 3-12. [Google Scholar]
- 36.Zecca, M., Basler, K. & Struhl, G. (1996) Cell 87, 833-844. [DOI] [PubMed] [Google Scholar]
- 37.Zecca, M., Basler, K. & Struhl, G. (1995) Development (Cambridge, U.K.) 121, 2265-2278. [DOI] [PubMed] [Google Scholar]
- 38.Burke, R. & Basler, K. (1996) Development (Cambridge, U.K.) 122, 2261-2269. [DOI] [PubMed] [Google Scholar]
- 39.Garcia-Bellido, A. C. & Garcia-Bellido, A. (1998) Int. J. Dev. Biol. 42, 353-362. [PubMed] [Google Scholar]
- 40.Martin-Castellanos, C. & Edgar, B. A. (2002) Development (Cambridge, U.K.) 129, 1003-1013. [DOI] [PubMed] [Google Scholar]
- 41.Simpson, P. & Morata, G. (1981) Dev. Biol. 85, 299-308. [DOI] [PubMed] [Google Scholar]
- 42.Adachi-Yamada, T. & O'Connor, M. B. (2002) Dev. Biol. 251, 74-90. [DOI] [PubMed] [Google Scholar]
- 43.De La Cova, C., Abril, M., Bellosta, P., Gallant, P. & Johnston, L. A. (2004) Cell 117, 107-116. [DOI] [PubMed] [Google Scholar]
- 44.Moreno, E. & Basler, K. (2004) Cell 117, 117-129. [DOI] [PubMed] [Google Scholar]
- 45.Jamora, C. & Fuchs, E. (2002) Nat. Cell Biol. 4, E101-E108. [DOI] [PubMed] [Google Scholar]
- 46.Landau, L. M. & Lifshitz, E. M. (1986) Theory of Elasticity (Pergamon, Oxford).
- 47.Goodwin, G. C., S. F. Graebe, M. E. Salgado. (2001) Control System Design (Prentice Hall, New York).
- 48.Wieschaus, E. (1978) Results Probl. Cell Differ. 9, 97-118. [DOI] [PubMed] [Google Scholar]
- 49.Milan, M., Perez, L. & Cohen, S. M. (2002) Dev. Cell 2, 797-805. [DOI] [PubMed] [Google Scholar]
- 50.Milan, M., Weihe, U., Perez, L. & Cohen, S. M. (2001) Cell 106, 785-794. [DOI] [PubMed] [Google Scholar]
- 51.Resino, J., Salama-Cohen, P. & Garcia-Bellido, A. (2002) Proc. Natl. Acad. Sci. USA 99, 7502-7507. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 52.Drasdo, D. (2000) Phys. Rev. Lett. 84, 4244-4247. [DOI] [PubMed] [Google Scholar]
- 53.Moreno, E., Basler, K. & Morata, G. (2002) Nature 416, 755-759. [DOI] [PubMed] [Google Scholar]
- 54.Bullions, L. C. & Levine, A. J. (1998) Curr. Opin. Oncol. 10, 81-87. [DOI] [PubMed] [Google Scholar]
- 55.Peifer, M. (1995) Trends Cell Biol. 5, 224-229. [DOI] [PubMed] [Google Scholar]
- 56.Tschumperlin, D. J., Dai, G., Maly, I. V., Kikuchi, T., Laiho, L. H., McVittie, A. K., Haley, K. J., Lilly, C. M., So, P. T., Lauffenburger, D. A., et al. (2004) Nature 429, 83-86. [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.