Abstract
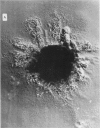
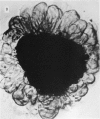
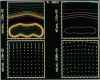
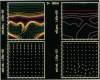
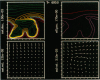
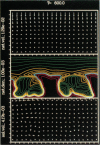
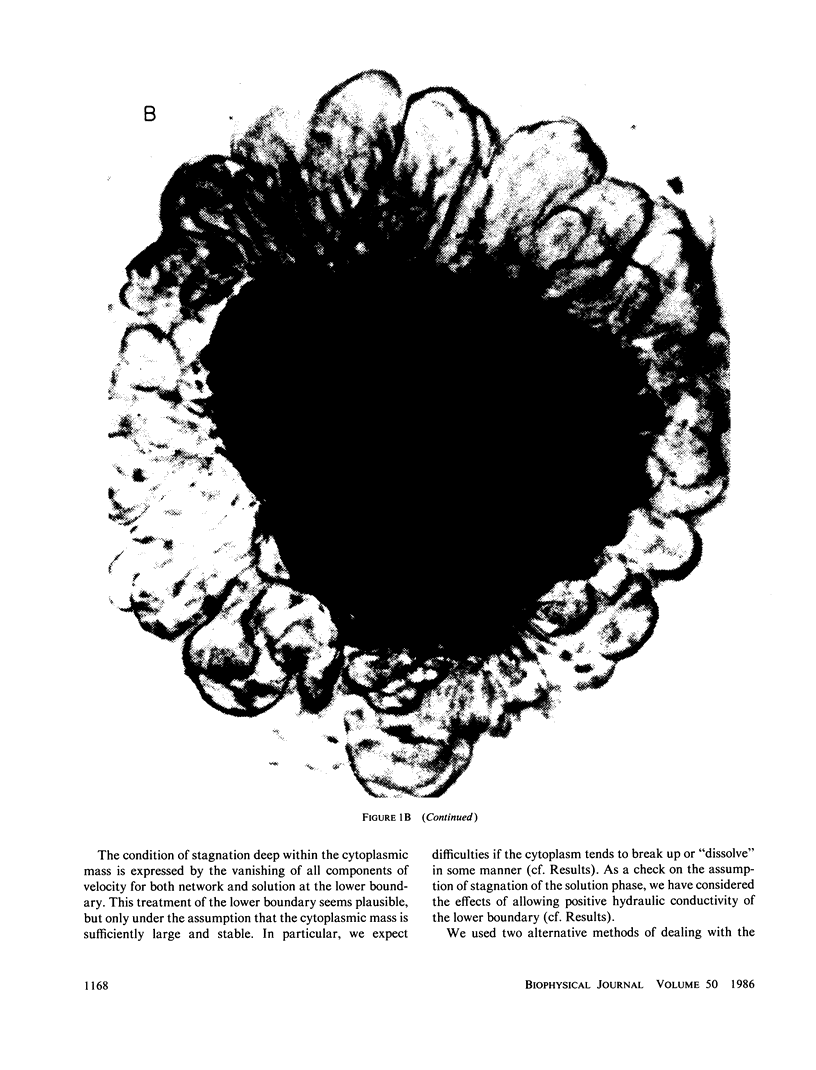
We stimulate the dynamical behavior of dissociated cytoplasm using the Reactive Flow Model (Dembo, M., and F. Harlow, 1986, Biophys. J., 50:109-121). We find that for the most part the predicted dynamical behavior of the cytoplasm is governed by three nondimensional numbers. Several other nondimensional parameters, the initial conditions, and boundary conditions are found to have lesser effects. Of the three major nondimensional parameters, one (D#) controls the percentage of ectoplasm, the second (C#) controls the sharpness of the endoplasm-ectoplasm boundary, and the third (R#) controls the topological complexity of the endoplasm-ectoplasm distribution. If R# is very small, then the cytoplasm contracts into a single uniform mass, and there is no bulk streaming. If R# is very large, then the cytoplasmic mass breaks up into a number of clumps scattered throughout the available volume. Between these clumps the solution undergoes turbulent or chaotic patterns of streaming. Intermediate values of R# can be found such that the mass of cytoplasm remains connected and yet undergoes coherent modes of motility similar to flares (Taylor, D.L., J.S. Condeelis, P.L. Moore, and R.D. Allen, 1973, J. Cell Biol., 59:378-394) and rosettes (Kuroda, K., 1979, Cell Motility: Molecules and Organization, 347-362).
Full text
PDF





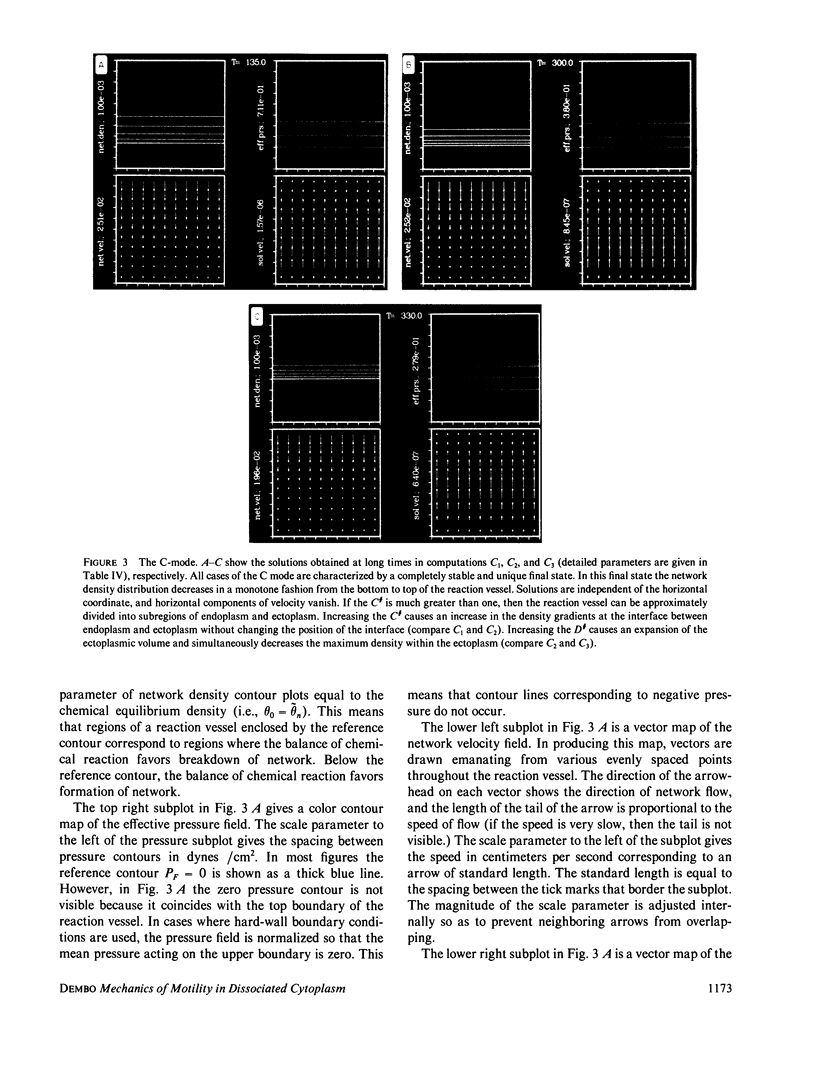

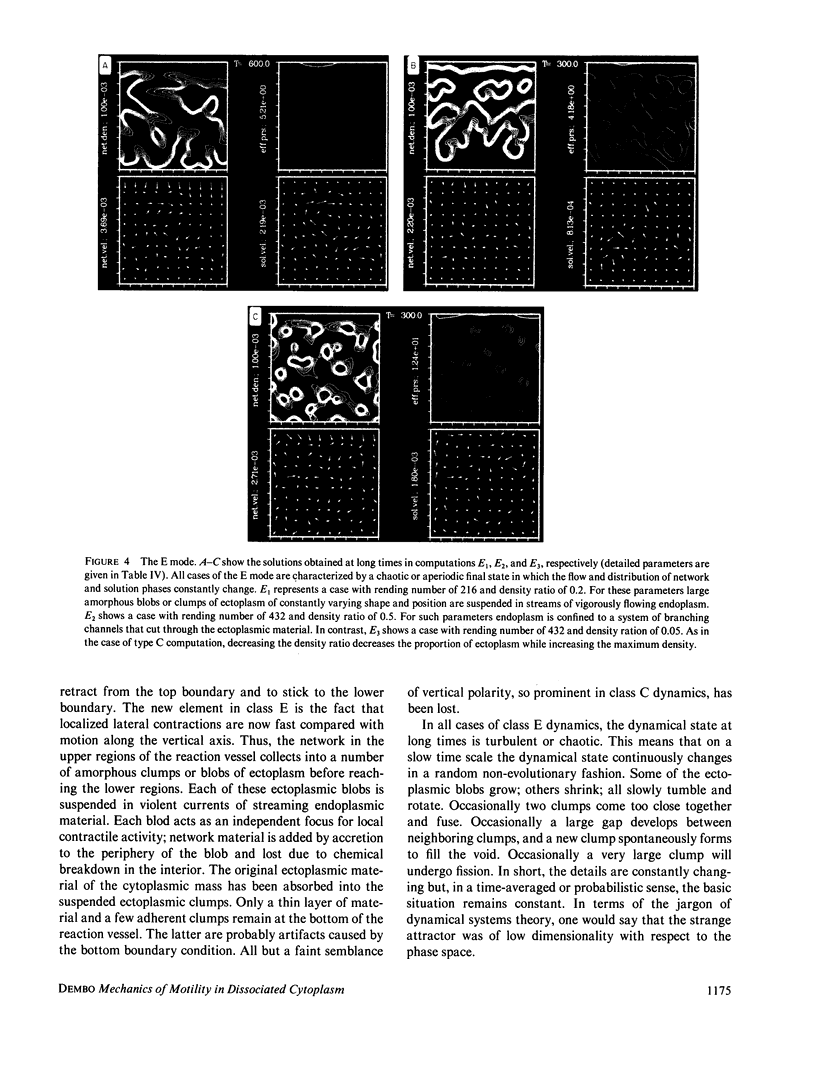
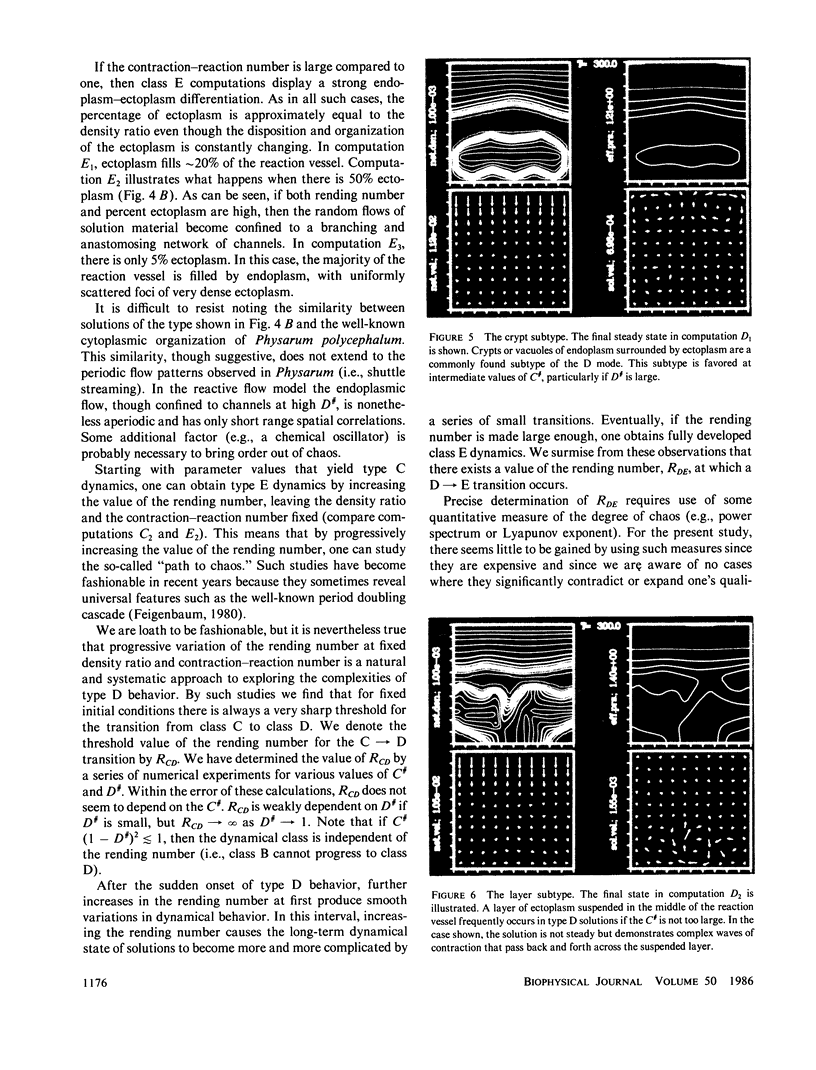
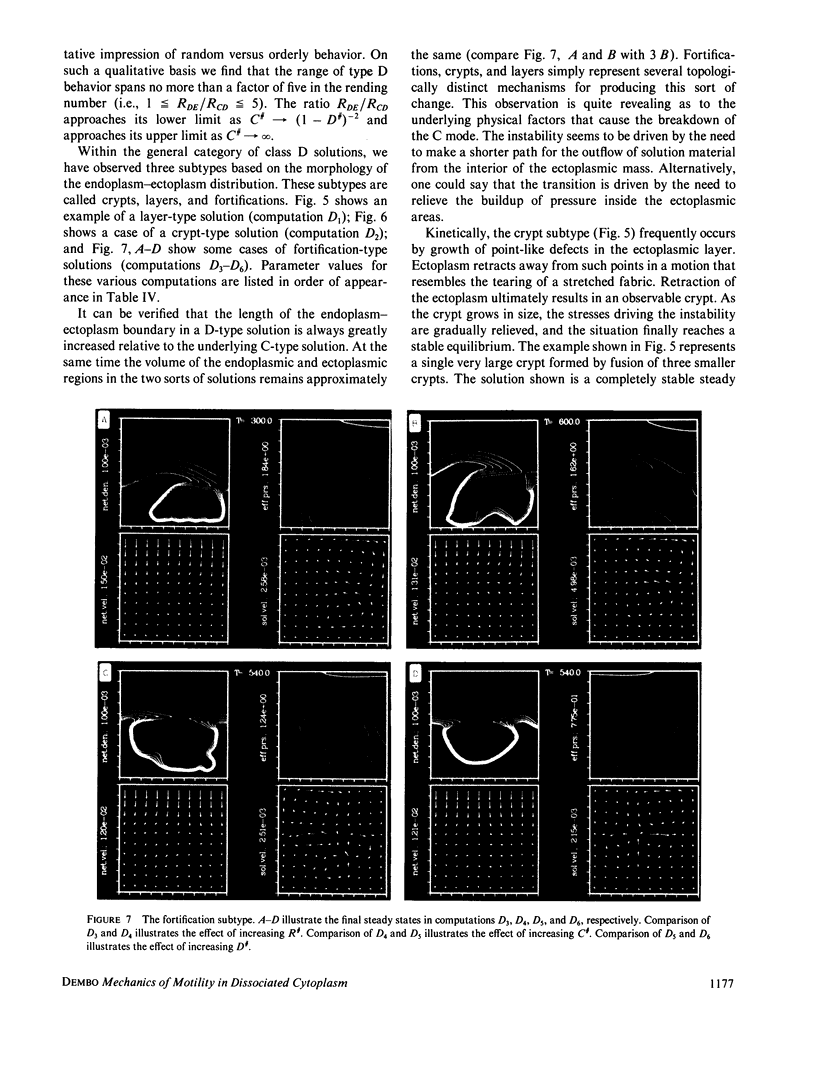

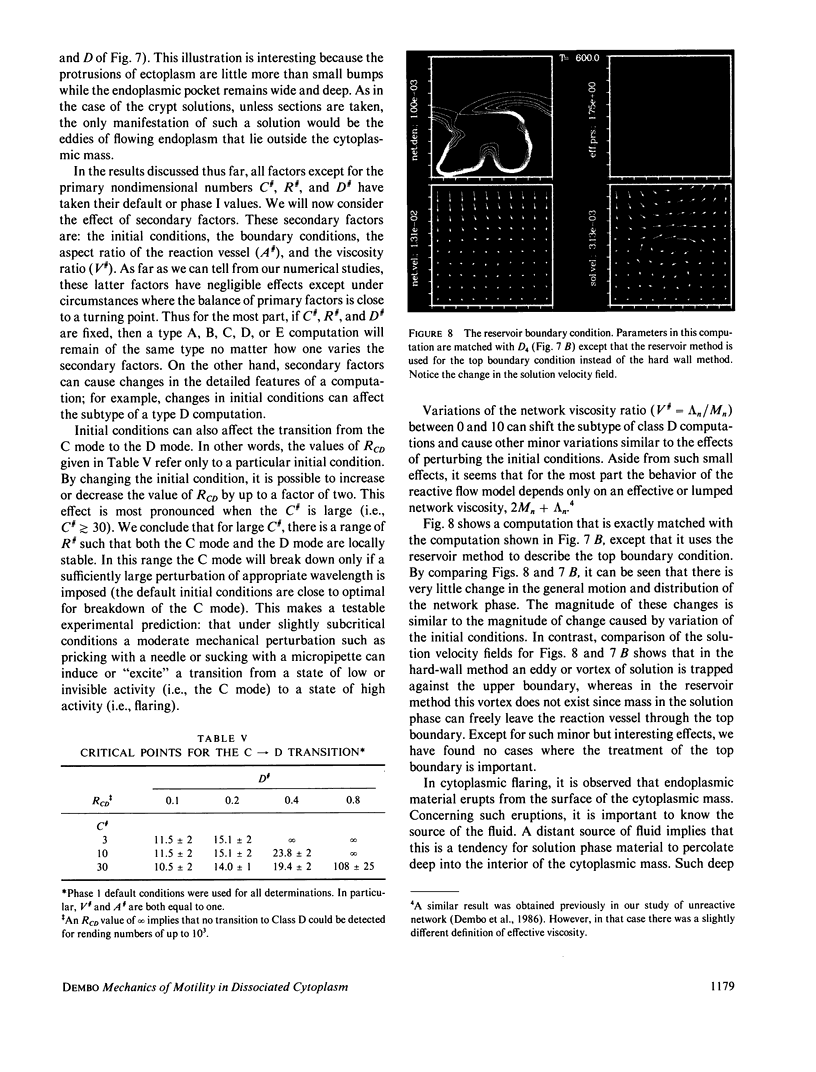
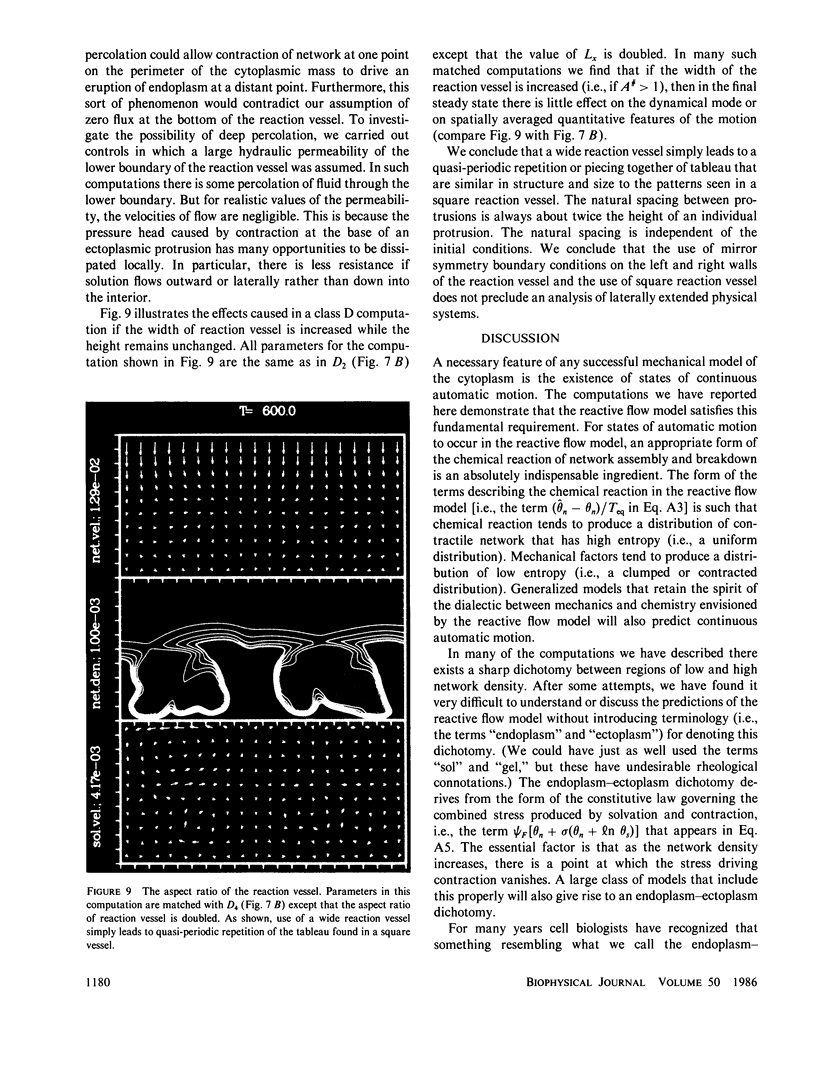



Images in this article
Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- ALLEN R. D. A new theory of ameboid movement and protoplasmic streaming. Exp Cell Res. 1961;Suppl 8:17–31. doi: 10.1016/0014-4827(61)90337-8. [DOI] [PubMed] [Google Scholar]
- ALLEN R. D., COOLEDGE J. W., HALL P. J. Streaming in cytoplasm dissociated from the giant amoeba, Chaos chaos. Nature. 1960 Sep 10;187:896–899. doi: 10.1038/187896a0. [DOI] [PubMed] [Google Scholar]
- Dembo M., Harlow F. Cell motion, contractile networks, and the physics of interpenetrating reactive flow. Biophys J. 1986 Jul;50(1):109–121. doi: 10.1016/S0006-3495(86)83444-0. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Dembo M., Maltrud M., Harlow F. Numerical studies of unreactive contractile networks. Biophys J. 1986 Jul;50(1):123–137. doi: 10.1016/S0006-3495(86)83445-2. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Higashi-Fujime S. Active movement of bundles of actin and myosin filaments from muscle: a simple model for cell motility. Cold Spring Harb Symp Quant Biol. 1982;46(Pt 1):69–75. doi: 10.1101/sqb.1982.046.01.010. [DOI] [PubMed] [Google Scholar]
- Pollard T. D., Ito S. Cytoplasmic filaments of Amoeba proteus. I. The role of filaments in consistency changes and movement. J Cell Biol. 1970 Aug;46(2):267–289. doi: 10.1083/jcb.46.2.267. [DOI] [PMC free article] [PubMed] [Google Scholar]
- THOMPSON C. M., WOLPERT L. THE ISOLATION OF MOTILE CYTOPLASM FROM AMOEBA PROTEUS. Exp Cell Res. 1963 Oct;32:156–160. doi: 10.1016/0014-4827(63)90078-8. [DOI] [PubMed] [Google Scholar]
- Taylor D. L., Condeelis J. S., Moore P. L., Allen R. D. The contractile basis of amoeboid movement. I. The chemical control of motility in isolated cytoplasm. J Cell Biol. 1973 Nov;59(2 Pt 1):378–394. doi: 10.1083/jcb.59.2.378. [DOI] [PMC free article] [PubMed] [Google Scholar]